An alternative to the production of transgenic animals as a means of conserving endangered species is the establishment of small populations as so-called domestic animal zoos, or the conservation of residual populations kept by breeders or organizations. The decisive point is to guarantee that these animals will constitute a reproductive community even if they are kept at different locations. This means that at least male animals will have to be exchanged between different herds, preferably according to an established breeding scheme.
The probability of survival of a small population may be estimated by means of mathematical models which also take into consideration such important variables as female fertility, vitality of calves, sex ratio, breeding plans etc. Appropriate analyses demonstrate that the extinction of small populations is inevitable, with female fertility being the most vulnerable factor (Senner, 1980; Yamada and Kimura, 1984). Yamada and Kimura (1984) have established a simulation model with variable population sizes, starting with the assumption that all individuals having reached breeding age will also be used for breeding purposes. This analysis has demonstrated that a population consisting of five or less animals will definitely be extinct even if all female animals having reached the reproductive age are used for breeding (Figure 26).
Further analysis by Yamada and Kimura (1984) has resulted in the following recommendations for conserving genetic resources:
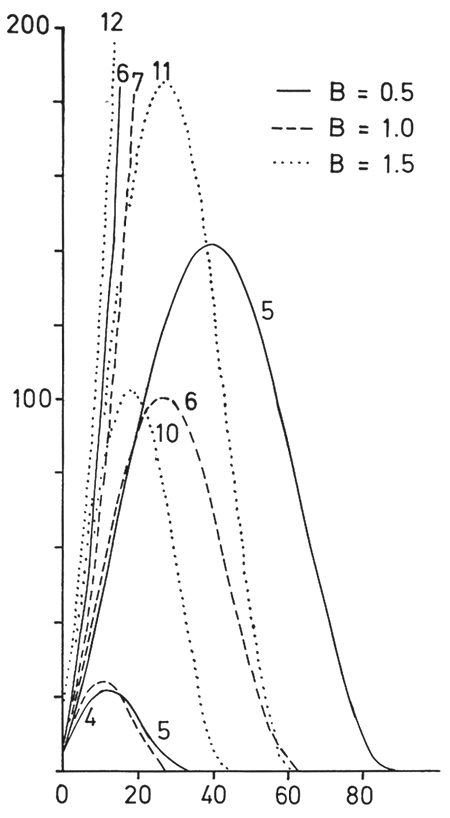
Figure 26: Number of Generations before Extinction of Small Populations in Relation to the Number of Founder Animals.
B = coefficient of regression of the negative logarithm of the fraction of offspring reaching reproductive age (According to Yamada and Kimura, 1984).
Gene frequencies in small populations may be subject to random alterations. The inheritance of a gene by the next generation is more or less a random process. If the population size is large these random events will be equalized due to the large number of matings and offspring. In very small populations shifts may occur if either a relatively small, or a large fraction of the limited number of offspring has received a certain gene. In the next generation a frequently occurring allele may again be inherited randomly to a larger or lesser extent. If alleles are randomly increased or decreased this may lead to the fixation of genetic loci (gene frequency = 1.0) or to the loss of an allele (gene frequency = 0.0). This process terminates variations at the affected gene locus.
If small populations are bred for many generations fixation or loss of an increasing number of genetic loci becomes inevitable thus leading to an increased level of homozygosity.
Eventually genetic drift is the result of accumulation of the effects of random variations in small populations. A number of examples demonstrate the effects of genetic drift in small populations of farm animals (Pirchner, 1979).
If the breeding lines remain small the effects of random drift are retained. The variance, δq2, between the gene frequencies of this population may be estimated by the equation given by Prichner (1979):
| δq2 = pq (1-(1-2N-1)t) | (5) |
with p representing the gene frequency (p + q = 1), N representing the population size, and t the number of generations.
Values for alterations of the variance of gene frequencies with respect to population sizes, gene frequencies and number of generations are shown in Table 8.
| Population size (N) | ||||||||
| 2 | 5 | 10 | 100 | |||||
| Gene frequency (p) | .5 | .1 | .5 | .1 | .5 | .1 | .5 | .1 |
| Generation (t) | ||||||||
| 1 | .063 | .022 | .025 | .009 | .012 | .004 | .001 | .0004 |
| 2 | .109 | .039 | .04 | .017 | .024 | .009 | .002 | .0009 |
| 3 | .144 | .052 | .068 | .024 | .036 | .013 | .004 | .001 |
| 4 | .171 | .062 | .086 | .031 | .046 | .017 | .005 | .002 |
| 5 | .191 | .069 | .102 | .037 | .057 | .020 | .006 | .002 |
| 10 | .235 | .085 | .136 | .059 | .100 | .036 | .012 | .004 |
| 100 | .249 | .089 | .249 | .089 | .249 | .089 | .099 | .035 |
| .25 | .09 | .25 | .09 | .25 | .09 | .25 | .09 | |
Table 8: Random Fluctuations of Gene Frequencies
The probability for a certain gene to be retained depends on the population size and on whether the gene in question is neutral or possesses selective value. As shown in Table 9 genes with selective values are almost always retained in large populations. In contrast, the probability of such genes to be retained in small populations is greatly reduced.
In order to keep drift variances as small as possible when embryo banks are established the embryos should be obtained from as many different matings as possible. This would also guarantee that the genetic differences between basic breeding populations and the embryo bank would be as small as possible. Storage of embryos is a very effective process since no genetic drift and hence no increase in inbreeding occur during storage.
Genetic drift variance during the establishment of embryo banks can be calculated according to Smith (1977, 1984 a, b) from the genetic variance and the numbers of parents and offspring:
| Vd = VG (nd + n + 2)/4 nds | (6) |
| Population size (N) | ||||||||||||
| 2 | 5 | 10 | 100 | |||||||||
| Gene frequency (p) | .5 | .1 | .01 | .5 | .1 | .01 | .5 | .1 | .01 | .5 | .1 | .01 |
| Selection coefficient (t) | ||||||||||||
| .5 | .88 | .34 | .04 | .99 | .63 | .10 | 1.0 | .86 | .18 | 1.0 | 1.0 | .86 |
| .1 | .60 | .14 | .01 | .73 | .21 | .02 | .88 | .34 | .04 | 1.0 | .98 | .33 |
| .01 | .51 | .10 | .01 | .52 | .11 | .01 | .55 | .12 | .01 | .88 | .34 | .04 |
| .001 | .50 | .10 | .01 | .50 | .10 | .01 | .50 | .10 | .01 | .55 | .12 | .01 |
Table 9: Probabilities for Gene Fixation
with Vd representing the drift variance, VG the genetic variance, s the number of unrelated sires, d the number of dams per bull, and n the number of progeny per dam.
Table 10 lists all drift variances occurring in embryo banks.
| Drift variance (x VG) | ||||||||
| Cows per bull | 2 | 4 | 20 | 100 | ||||
| Progeny per cow | 1 | 5 | 1 | 5 | 1 | 5 | 1 | 5 |
| Bulls | ||||||||
| 2 | .31 | .21 | .22 | .17 | .14 | .13 | .13 | .13 |
| 4 | .16 | .11 | .11 | .08 | .07 | .07 | .06 | .06 |
| 20 | .03 | .021 | .022 | .017 | .014 | .013 | .013 | .013 |
| 100 | .006 | .004 | .004 | .003 | .003 | .0027 | .0026 | .0025 |
Table 10: Drift Variance in Embryo Banks (Values to Be Multiplied by VG)
The values in Table 10 are factors which must be multiplied with the initial variance VG. The number of progeny per dam depends on the number of conserved embryos that have been conserved from this dam and the survival rate of these embryos after thawing and transfer to suitable recipients.
The values demonstrate that drift variance may be reduced especially by conserving embryos from as many different matings as possible. The contribution of increasing the number of dams per bull and of the number of progeny per dam reduces drift variance only insignificantly. If more than four dams per bull are used drift variance is almost identical for 5 or 10 offspring.
One disadvantage of embryo banks is the fact that investigations cannot be carried out at all times because animals are not available. Another disadvantage is that reactivated populations are maintained, with respect to performance traits, at the level achieved at the time of freezing, because of the lack of further breeding successes during storage. However, this point may not be a disadvantage.
Embryos used for cryoconservation should constitute a collection representative for the population. The number of embryos required depends upon the success rates that can be achieved by transfer of thawed embryos. At least 25 unrelated dams and sires should be available as parent animals.
Qualitative traits are determined by single genes. If one has to fall back upon qualitative traits during the activation of genetic resources the introduction of the corresponding gene(s) depends upon the nature of the genetic resources.
An important factor in establishing genetic resources is the probability with which a particular gene is conserved. It is particularly important to pay attention to factors governing this probability in order to guarantee a high chance of individual genes being conserved. The following sections will demonstrate that these factors depend upon the way in which genetic resources are established.
The probability of a particular gene of a population not being present in a gene library depends upon the gene frequency and the size of the genomic library.
If the gene frequency in the starting population is represented by q, the probability, p1, that this gene is missing in N bulls of a semen bank is
| p1 = (1-q1)2N1 | (7) |
With more than 10 embryos per mating the probability of a gene not being present in the genome reserve is approximately (Smith, 1984 a):
| p1' = (1 - q1)4N1 | (8) |
As shown in Table 11 the probability of a particular gene not being present in a genetic reserve is rather low.
A complete genomic library containing every conceivable gene at least once consists of at least several hundred thousands clones (Table 12).
The conservation of genes in genomic libraries (See Section 3.2.1) allows the probability of a particular genomic DNA sequence being contained in this library to be estimated.
The number of required clones can also be estimated for cDNA libraries. A typical mammalian cell contains up to 30000 different mRNA sequences. Williams (1981) has estimated the number of clones required for a representative cDNA library from fibroblasts which contain approximately 12000 different mRNA sequences. Rare mRNA sequences which are only represented in less than 14 copies per cell constitute approximately 30 percent of the entire mRNA and consist of ca 11000 different mRNA molecules. This means that approximately 37000 clones (i. e. 11000/0.30) will be required to ensure that all rare mRNA molecules are represented in the library. If this value is used for the equation given above (n = 1/37000) it follows that the entire cDNA library must contain approximately 170000 clones to ensure a probability of p = 0.99%.
| Gene | No. of conserved genotypes | Probability of not being contained in a | |
| semen bank (p1) % | embryo bank (p1) % | ||
| .5 | 1 | 25 | 6.3 |
| 2 | 6.3 | .39 | |
| 10 | 9.5×10-5 | 9.1×10-11 | |
| 100 | 6.2×10-59 | 0 | |
| .3 | 1 | 49 | 24 |
| 2 | 24 | 5.7 | |
| 10 | 8.0×10-2 | 6.4×10-5 | |
| 100 | 1.0×10-29 | 1.1×10-60 | |
| .1 | 1 | 81 | 66 |
| 2 | 66 | 43 | |
| 10 | 12 | 1.5 | |
| 100 | 7.1×10-8 | 5.0×10-17 | |
| .01 | 1 | 98 | 96 |
| 2 | 96 | 92 | |
| 10 | 81 | 67 | |
| 100 | 13 | 1.8 | |
| .001 | 1 | 99.8 | 99.6 |
| 2 | 99.6 | 99 | |
| 10 | 98 | 96 | |
| 100 | 81 | 67 | |
Table 11: Probability of a Particular Gene Not Being Present in a Genome Reserve
Table 12 is a compilation of values indicating the number of clones that are required for a particular DNA or cDNA sequence to be represented in a corresponding library with a given probability.
So as to estimate the probability of a particular gene of a population being contained in a library it is necessary to take into consideration the probability of individual animals used for establishing the library as carries of this particular gene (See Table 8). For rare genes this means that it will be absolutely essential to establish libraries from several animals.
| Probability % | No. of DNA clones ×105 | No. of cDNA clones × 105 |
| 90.0 | 4.1 | .9 |
| 95.0 | 5.3 | 1.1 |
| 99.0 | 8.1 | 1.7 |
| 99.5 | 9.4 | 2.0 |
| 99.9 | 12.2 | 2.6 |
| 99.99 | 16.3 | 3.4 |
Table 12: Number of Clones Required to Ensure that a Particular DNA or cDNA Sequence is Represented in a Corresponding Library with a Desired Probability.
Many traits that are of interest if genetic resources are activated are quantitative in nature. In contrast to qualitative traits quantitative traits are usually the result of an interaction of many genes or combination of genes. It is therefore more or less futile to attempt to restore such traits from conserved individual genes.
However it may be possible to obtain such polygenic traits if entire genomes have been conserved and reactivated although it may not be possible to exploit these genetic combinations in a form isolated from the residual genome.
In order to obtain genes coding for quantitative traits from a genetic resource and to transfer them into a currently available population, controlled crossbreeding is required.
As far as quantitative traits are concerned chances are low for the controlled short-term exploitation of a conserved breed. However, the application of molecular biological techniques should allow characterization of individual genes and even gene combinations responsible for polygenic traits in the future, which would significantly improve the current situation.
Nevertheless, severe future losses must be expected with respect to genetically complex traits such as fertility, disease resistance, or vitality. In such cases the ability to resort to genetic resources would be of valuable help. It has already been mentioned that the reintegration of desired gene combinations responsible for quantitative traits in conserved breeds must be effected by crossbreeding into currently available breeding populations. This means that especially in view of costs the conservation of semen should be considered as the method of choice.