G. Jolánkai
Research centre for Water Resources Development
Budapest, Hungary
1. INPUT LOAD DETERMINATION AND ECOSYSTEM MODELLING IN THE DESIGN AND CONTROL OF PERFORMANCE OF FISH FARMS
2. WATER QUALITY MANAGEMENT PRINCIPLES AND SYSTEMS VIEWING
3. INPUT LOAD DETERMINATIONS
4. AQUATIC ECOSYSTEM MODELS
5. SUMMARIZING REMARKS
6. REFERENCES
Although the mathematical modelling of aquatic ecosystems is not yet a common tool in the design and operation of fish farms, the knowledge and quantitative evaluation of a system (the sources of nutrients and their fate along the food chain) could, even today, provide for a better understanding of the processes that are taking place. This knowledge and understanding may be the basis of exercising control over the system that could provide for more efficient performance, the prediction of emergency situations such as oxygen depletion and algal blooms, and the saving of fodder costs. These are only a few of the probable advantages. As a general rule, the description of a system using quantitative terms, and an investigation of the cause and effect or input-response relationships and interactions (instead of relying only on qualitative data) will give more possibilities to the operator in controlling this system.
To elucidate these general statements let us examine a common situation. Due to increased input of nutrients and organic matter, combined with high temperatures, the dissolved oxygen content of water may drop drastically, resulting in severe effects such as fish kills. If one has a validated model that describes oxygen depletion as a function of the organic matter present in the water and sediment, the photosynthetic and respiration activity of algae, the wind velocity, the temperature and other affecting factors, then one may be able to predict such situations in advance and take the necessary counter measures of aeration, removal of organic sediment and decrease of external load. On the contrary, if one only knows by qualitative experience that such situations may be developing on hot and windless days, but does not know the other causes and interrelationships in quantitative terms, then one's action may be too early or too late, or entirely ineffective.
This does not mean, of course, that experience is not of vital importance. Without experiments and field measurements the best mathematical model will be only a mere game to play.
These notes do not intend to give full details of ecosystem modelling, since this could be the subject of two or three semesters and not three lessons. They are intended to give a brief introduction into the basic principle of such system viewing and model developments, on the basis of which those interested may be able to start to read the relevant literature and to develop their respective models gradually, using their own measurement data.
Man, the water user, and the water that is available for the user, form the main components of an ever continuing interactive cycling process, both in terms of water quality and quantity (Figure 1). With his uses, man alters the quality and quantity of the water resources and their allocation which, in turn, alters man's activity. Thus, altered availability of water, in terms of quantity and quality, both in time and space, will induce man to alter his activities to get better and more water during periods of former shortage, and also in places where there has not previously been water.
This refers to any water uses and the same principles of management should be followed (Figure 2). Having set the objectives, availability and qualitative composition of the water should be defined, providing a data base. Next comes the planning of water utilization facilities, and one has to predict their effect both in terms of quality and quantity. Following implementation and a continuing monitoring, one has to control the performance of the altered system, and this goes on and on for ever. In the core of all these processes there is, or at least there should be, a basic tool; a system model that provides simulation and prediction capabilities. On the basis of this, one is able to tell with some accuracy what will be the result of his planned activities or his management strategies.
For a regional water management plan and operation, these briefly described processes may form a very complex system, in the core of which there should be a computer that houses a complex and ever refined data bank as well as all the models.
Figure 1. Water cycling process
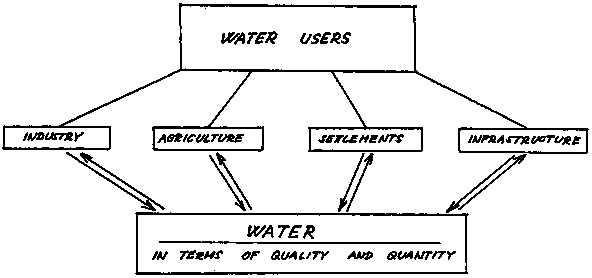
Figure 2. water management system
On a smaller scale, however, such as the planning, operation and maintenance of an impoundment for aquaculture, objectives are clearly defined by the volume of water needed and by the quality that is required. Monitoring could be confined to a couple of observation points, measuring discharge, and a well defined list of water quality parameters selected as a function of the water use concerned. Inputs and outputs in terms of water and water quality are measured. But, in addition, selected parameters that describe conditions within the system, the so-called "state variables", are also measured. Models for design and operation can be simplified to some extent. For example:
- a rainfall runoff model to predict the inflows- a storage model to describe the change of water volumes in the impoundment, or to provide a basis of regulating the outlet, and
- a water quality model that describes the changes of the state variables within the impoundment.
Management strategies are also relatively well definable. They consist of two main groups of activities:
(a) Control of the input and output.
(b) Control exercised within the system.
The first group encompasses such activities as the regulation of inflow and outflow, the control of pollution sources on the watershed, and the dosage of fodder for fish feeding if a fish pond is being used. Such alternatives as release of water from the upper or from the lower layers belong also to this group. Generally speaking, the control of everything in terms of quality and quantity that gets into the system, or out of the system, belongs here.
In-lake control activities, such as artificial aeration, dredging, removal or planting of macrophytes, etc. belong in the second group (see Figure 3).
The sources of materials that enter a given water body, whether it be an impoundment or a pond may be grouped into the following categories (Figure 4):
(a) Direct point sources such as an effluent discharge.(b) Indirect point sources such as effluent discharges on the watershed that is drained into the water body.
(c) Direct non-point sources such as input from the atmosphere, or via surface or sub-surface runoff.
(d) Indirect non-point sources such as diffuse sources of materials on the watershed that enter the given water body via the inflowing stream. For example, fertilizers belong to this group.
Pollutants and other materials not necessarily harmful to aquatic life originating from these sources may significantly alter the aquatic ecosystem. Some examples are:
- toxic substances may partly or fully kill living things, thus causing severe disturbance, or even total breakdown, of the system.- nutrients such as phosphorus and nitrogen originating from domestic sewage and fertilizers are important factors in an aquatic ecosystem, having effects throughout the food chain.
- organic matter originating from sewage and from natural sources is decomposed by bacteria which, in turn, use the dissolved oxygen of the water, thus depleting oxygen levels.
Figure 4. Sources of water management
As a generalization, it may be stated that almost any substances that enter the system from the outside have potential to alter the system.
It follows from the above statements that for the establishment of any control or management strategies, a knowledge of the inputs has to be gained in terms of both quality and quantity.
As a general rule, all sewage inputs in the catchment should be measured with due regard to diurnal and seasonal variations, both in terms of discharge and quality. The main concern should be focused on the determination of pollutant loads entering the system via the inflowing stream(s). In the case of a smaller impoundment surrounded by dikes, the effects of direct non-point source input can be neglected.
The most straightforward solution to the problem of pollutant loads entering the system is to establish a continuous monitoring station directly upstream of the intake consisting of:
- a hydraulic structure such as a weir or venturi flume that enables discharge determination, equipped with a continuous water level recorder;- an automatic sampler that takes water samples from the stream at regular intervals, including rising and falling floods.
If this equipment is installed then mass flux of materials (load) can be defined for any point of time as
L = Q × C
where
L = the mass flux (load) of the material concerned in mass per unit of time (i.e. g/sec, kg/day, tons/year, etc.)Q = the discharge of the stream (in m3/sec)
C = the concentration value of the material concerned in mass per unit volume dimensions (i.e. g/m3, mg/l, etc.)
The calculation can be for any period of time as
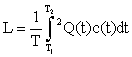
where T = T2 -T1 is the length of the period of time concerned.
However, what one really needs is a prediction of load conditions. For this, the following methods can be used:
(i) time series analysis, to be followed only when records of reasonable length such as 4 to 5 years are available(ii) a rainfall runoff model can be coupled with regression models developed between flow rate and concentration or flow rate and load values, as indicated in Figure 5.A
(iii) other non-point source runoff models may be calibrated using observation data (Jolánkai, 1983, Novotny, 1980).
Flow versus concentration and flow versus load regressions have other applications too. When flow records exist but an automatic water sampler is not available (which is frequently the case), the flow series can be converted into load series (Figure 5.B) if reliable load versus flow relationships exist. It should be noted that the actual relationship between flow and load varies according to the parameter being measured or according to many other factors such as seasonal variations, land use activities, application of agricultural chemicals, etc. Thus, the relationship is not bivariate but multi-variate and, consequently, should be developed in such a manner as to allow for seasonal variations and for rising and falling water level differences as indicated in Figure 5 A and B.
Figure 5. Rainfall-load and flow-load models
Regressions of these types should be developed by plotting all the available flow and load data pairs on a sheet, then separating data corresponding to different seasons, flood waves, and base flows. The type of the regression curve should then be selected (i.e. exponential, logistic, power, logarithmic, linear, etc.). Note that it is desirable to carry out regression analysis using different theoretical curve, equations and then to select the one that gives the best fit.
There may be an additional problem of input load determination if the main polluting source is somewhere far upstream on the watershed. In this case the removal of pollutants at the source may only result in a lessened decrease of input load at the reservoir or pond intake. In such cases, longitudinal water quality profile studies of the stream in question should be performed, and the data analysed by a water quality model that describes the transport and transformation processes along the stream. This problem is briefly outlined in Figure 6.
The corresponding water quality model considers the following:
- transport of the material caused by turbulent dispersion- transport of the material caused by flow itself (convective transport)
- internal transformation of the material (degradation, settling, uptake by organisms, decay, scouring from the bottom, etc.)
- external sources of the material.
The corresponding mathematical model is shown in a generalized form in Figure 6.
Actual application of such models is usually made in a much simplified form: for example, neglecting dispersion terms and considering only longitudinal transport and a decay term of first order kinetics type, one may end up with an equation as simple as:
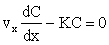
Assuming steady state conditions, this under initial conditions C = Co at x = 0 can be integrated resulting in a simple exponential decay profile of the type
where
C is the concentration of the material (in mass per volume dimensions, i.e. g/m3)vx is the longitudinal flow velocity in length per time dimension (i.e. m/sec)
K is the decomposition (decay or other loss) rate coefficient in time-1 dimensions (i.e. day-1)
Co is the initial concentration downstream of the pollution source in question, computed as:
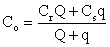
where
Cr and Cs = the concentration of the substance in the river upstream and in the effluent discharge respectively, andQ and q are river and effluent flow rates respectively.
Note, however, that models could be much more complex in the case of an actual problem, where the effects of interacting quality components, internal sources and sinks, etc. should be considered. For more details consult the works of Velz (1970), Tohomann (1972), Jolánkai (1976), Jolánkai (1979), Chen and Orlob (1972), Jörgensen (1983).
These are the models that would describe the response of the system to inputs as discussed above (see also Figure 3).
The basic theoretical principle behind these models is the same as was discussed before. The main differences in the case of a smaller pond or lake are that dispersion and convective transport of the materials can be neglected, thus assuming the principle of a fully mixed reactor (Chen and Orlob, 1972). Thus, transformations of the substances and their cycling within the components of the ecosystem should be described as a function of time, and a function of other (usually time dependent) factors.
Taking a closer look at the ecosystem, one may end up with a schematic representation of the complex processes such as shown in Figure 7. It should be noted that
- such a systems approach is always an idealisation and simplification of the reality, and- this simplication should focus on the selection of components (state variables) that are important in the system in question, and the quantification of which can be assured on the basis of real measurements.
Before entering into a discussion of somewhat more detailed system interactions, let us examine the basic principles of model building. Consider an ecosystem that is described by five state variables A, B, C, D, E, as shown in Figure 8. Assume the following:
- They are represented by their concentration, that is, mass per unit volume of water (or rather by the concentration of a single element forming part of their mass, for example a nutrient) to avoid the use of conversion factors for the time being.- A is the only component that receives an external input in the form of LA.
- A and B are interexchangeable (for example, scouring and settling of a material)
- A will be taken up by C for its growth and C will be a food for D
- Both C and D will decay into E
- E will convert into B
- Components in the water body (A, C and D) will flow out of the system at a rate of Q
- Assuming first order reactions in all of these processes (which is nonsense in reality), the system processes can be described by the equations shown in Figure 8.
It should be noted that all terms in the equations are in concentration per time dimension. Consequently, external loads like LA, are expressed as the load value divided by the lake volume, and outflow rate is expressed as the flow rate divided by the lake volume. For example, outflow rate in m3/day divided by lake volume in m3 will result in specific outflow rate of day dimension. In a similar manner, reaction rate coefficients are also expressed in time-1 dimensions.
Figure 7. Schematic representation of an aquatic ecosystem (after Chen and Orlob)
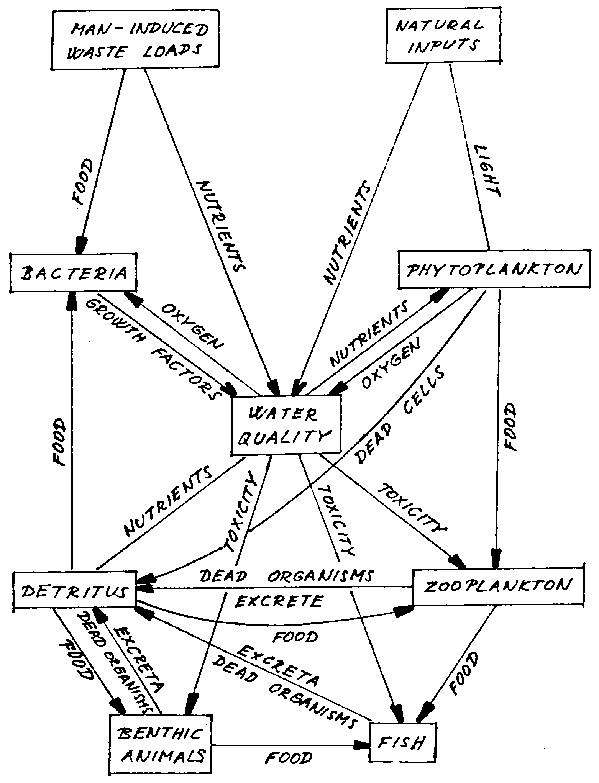
Figure 8. Fictitious ecosystem representation to explain model building rules
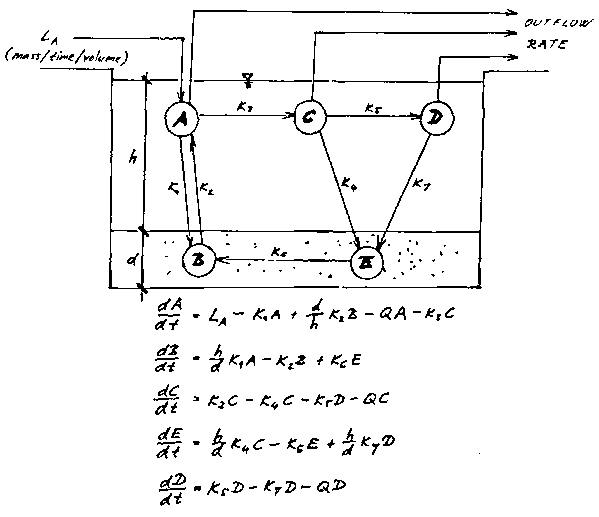
As general rules for checking the mathematical correctness of the model equations one should keep in mind that:
- all terms in model equations should be in the same dimension- the number of model differential equations equals the number of state variables
- the number of terms on the right side of these equations equals the number of arrows pointing towards and away from components on the model diagram of the system (as shown on Figure 8).
Models of this type are usually solved by finite difference approximation methods, such as the Runge-Kutta approximation.
A somewhat more realistic representation of a lake's ecosystem is presented in Figure 9. Following the above principles of model building, the basic model system of this scheme would consist of 16 basic differential equations and a large number of subsidiary models that describe reaction rates, growth, predation, mortality, etc. , Models of a similar type can be found in the works of Jörgensen (1983), Chen and Orlob (1972), Park et al,(1975), Kelly (1973) and King (1973), to mention a few of the extensive works dealing with this subject. At this stage of model building, modellers have to consult the relevant literature and/or the expert in this field. Instead of presenting model equations some general considerations are given below:
(i) Material flows of abiotic components (neglecting dispersion and convection, i.e. assuming a fully mixed reactor) will be described as
|
Mass transfer = |
Input-Output + scouring - sedimentation |
|
|
± reaeration - decay or decomposition |
|
|
± chemical transformation ± biological uptake |
|
|
± respiration |
(a) Sedimentation is usually taken into consideration as a simple first order kinetic process where the settled mass of material is proportional to the material present in the water with a proportionality factor (k). Scouring (or uptake from the bottom compartments) can also be considered as a first order relationship, but in some cases effects of wind velocity should also be considered. In some cases diffusion across the sediment-water interface predominates in the processes and should be described with diffusion equations similar to the dispersion terms in Figure 6.(b) Reaeration across the water surface is usually taken into consideration as a first order kinetic term, where the reaeration factor K can be related to surface diffusion factors of depth, wind velocity, etc.
(c) Decay and chemical transformation processes are usually considered as first order kinetic terms.
(d) Biological uptake and respiration of abiotic substances are usually considered to be proportional to the growth and respiration of the biota (with constant conversion factors such as the amount or fraction of phosphorus in the algae biomass).
(ii) Flows of materials in the biotic compartments are usually described by the following four processes:
(a) Feeding (or nutrient uptake for plants), that is the uptake or ingestion of a material from another compartment.(b) Predation (when the flow is considered from the compartment being fed upon, the feeding is termed predation).
(c) Excretion (plus egestion) is the difference between the material taken into a compartment and that part of the ingested material applied to growth, respiration and death.
(d) Respiration.
It should be recognized that there is a physical limitation to the amount of material taken up by any organism or group of organisms per unit of time. This has been observed experimentally for uptake of nutrients by algae and has been shown to be true also for feeding animals. The most widely accepted mathematical form of this limitation is the so-called Michaelis-Menten or Monod type of kinetics described as
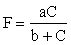
where F is the rate of transfer, a is the rate constant, b is the concentration at which half the maximum rate occurs (the half saturation constant) and C is the concentration of the material being eaten or taken up. Thus, the feeding rate of algae, zooplankton, fish and bacteria as a function of their food supply can be described by the above expression.
To convert this formulation (and some of the above statements) into mathematical terms one can construct a differential equation, for example for fish, as follows: (King, 1973).

where
|
F |
is the concentration of fish (grouped into various categories, such as cold and warm water zooplankton feeders, benthic animal feeders, etc.) |
|
KB1 |
is the biota activity rate adjustment coefficient (assumed to be temperature dependent). |
KB1 is found as follows:
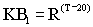
where
|
R |
is a temperature rate coefficient and T is the temperature in C |
|
FG |
is the fish growth rate at 20 C° |
|
FMAX |
is the maximum specific fish growth rate at 20 C° |
|
ZB |
is the quantity of zooplankton or benthic animals available for grazing |
|
ZB2 |
is the half saturation constant for fish grazing on zooplankton or benthic animals. That is, zooplankton concentration at which the fish in question achieve half of the maximum growth rate |
|
FM |
is the fish mortality rate at 20 C° |
|
FR |
is the fish respiration rate at 20 C° |
|
HARVEST |
is the rate of fish removed by fishing. |
It should be noted that this equation is only one of the sixteen equations related to Figure 9. Similar equations can be written for other biotic compartments (zooplankton, algae, etc.) and abiotic compartments (phosphorus, nitrogen, oxygen, etc.).
Note also that different factors may have to be considered in other compartments. For example, algae growth will be limited by light availability (where the self-shading effect also plays a significant role, and by the availability of phosphorus, nitrogen and carbon. All of these factors may be represented by monod kinetics. Wind, light and temperature correction factors have to be applied in many equations.
The main objectives of these notes are:
- to provide guidelines for the papers to be presented in this course.- to outline some basic principles of a systems approach to such problems as aquaculture design, management and operation.
- to illustrate with some simple examples the basic concepts of model building that can be used as tools for management and control of systems such as impoundments and fish ponds.
Chen, C.W. and G.T. Orlob, 1972, Ecologic simulation for aquatic environments, Walnut Creek, California, Water Resources Engineers Inc., (WRE 1-0500)
Jolánkai, G., 1976, The role of transversal mixing in the simulation models of water pollution control. Res. Water Qual. Water Technol. Ser.. Budapest
Jolánkai, G., 1979, Water quality modelling. In Vizminõség szábalyozás a Környezetvédelemben, edited by P. Benedek and P. Litheraty. Budapest, Mülszaki Könyvkiadó. (in Hungarian)
Jolánkai, G., 1983, Modelling non-point source pollution. In Application of ecological modelling in environmental management, edited by S.E. Jörgensen. Amsterdam, Elsevier Scientific Publishing Company
Jörgensen, S.E., 1983, Application of ecological modelling in environmental management. Amsterdam, Elsevier Scientific Publishing Company
Kelly, R.A., 1973, Conceptual ecological model of the Delaware estuary. In Systems analysis and simulation in ecology. Vol. 4, edited by B.C. Patten. Washington, D.C., Resources for the Future Inc.
King, I.P., 1973, A river basin ecological model. Walnut Creek, California, Water Resources Engineers Inc.
Novotny, V., 1981, Handbook of non-point pollution. New York, Van Nostrand Reinhold Co.
Park, A., 1975, A generalized model for simulating lake ecosystems. Publ. Environ. Sci. Div. Oak Ridge Natl. Lab.. Tenn., (691)
Thomann, R.V., 1972, Systems analysis and water quality management. New York, McGraw-Hill
Velz, C.J., 1970, Applied stream sanitation. New York, John Wiley and Sons Inc. 1970