by Howard S. Geller and Gautam S. Dutt1
1 We would like to thank Richard Schroeder, Tom Lavell and Edward Seliga for sharing their test data with us and Miriam Goldberg for providing advice regarding statistical analysis.
1. Introduction
2. Cooking practices
3. Measuring fuel consumption and efficiency
4. Simulation testing
5. Procedures for a series of cookstove performance tests
6. Interpretation of test data
7. Conclusions
A recent survey in a group of villages in South India revealed that the energy consumed for cooking in these villages amounts to 8.0 GJ/capita/year (ASTRA, 1981). This is comparable to the fuelwood demand for domestic cooking in other developing countries except where there is an extreme shortage of traditional fuels (Openshaw, 1978). By contrast, the average amount of energy consumed for cooking in the U.S. using gas stoves with pilot lights is 3.1 GJ/person/year (Lin et al., 1976). If the pilot light is eliminated, energy use for cooking on a gas stove would fall to 1-5-2.0 GJ/person/year. The much higher level of cooking energy consumption in villages in developing countries is chiefly a result of lower fuel economy.
One approach to alleviating fuelwood shortages is thus to reduce the demand for fuelwood by introducing more fuel efficient cookstoves and cooking practices. Efforts in this direction have, until recently, been unsuccessful for the most part (Joseph, 1979). However, many projects concerning the design and dissemination of more fuel efficient cookstoves and cocking practices have been initiated in the past few years.2 As a result, much has been learned regarding the performance of so-called improved cookstoves and the obstacles to introducing new stoves in developing countries.
2 A recent cookstoves newsletter lists 51 countries where work on improved cookstoves is being carried cut (Aprovecho Institute, 1981).
One lesson is that a successful programme for increasing cooking efficiency could require a number of activities including:
1. the design and development of improved stoves;
2. adapting a stove design to the local cooking needs and cultural conditions;
3. training people to build improved stoves;
4. ensuring that new stoves are being used properly;
5. promotion of the new stoves.
The evaluation of fuel consumption and/or fuel efficiency is likely to be an essential element in the above activities. For example, measurement of fuel consumption or efficiency is needed to design more efficient stoves and to compare stoves (item 1 above), and to determine fuelwood savings under particular operating conditions (items 2 and 4).
Information regarding fuel consumption and energy efficiency during cooking may be useful to individuals other than developers and promoters of improved stoves. For example, government officials could use reliable estimates of fuelwood consumption to forecast future energy demand and to plan fuel substitution programmes. Forestry officials may want to estimate fuelwood demand in order to plan an afforestation project. In addition, rural development specialists may want to encourage the use of improved stoves or cooking practices which are relatively fuel efficient as part of larger development programmes. The evaluation of cookstove performance within a fuelwood survey should provide data which is useful to those concerned solely with improved stoves as well as to those with broader interests.1
1 In some developing nations, cooking over an open fire is widely practised. The term "stove" and the methods for evaluating cookstove performance as described in this paper also apply to open fire cooking,
This paper describes the methods for evaluating cooking fuel economy during a fuelwood survey in developing countries. In section 2, we briefly summarize the qualitative information pertaining to cooking which should be collected along with performance data. The principles for measuring fuel consumption, energy consumption, and efficiency during cooking are presented in section 3. Section 4 describes the methods for testing stove performance in the laboratory under conditions simulating cooking. In order for test results to be meaningful, the measurements must be part of a carefully designed testing procedure. Factors to consider when planning a series of tests are presented in section 5 along with a specific procedure for organizing a set of comparative tests. In section 6, the analysis and interpretation of test results are discussed using examples from India and Upper Volta.
A survey of cooking conditions and practices should precede or accompany tests of fuel economy. There are several reasons why such a survey is valuable. At a practical level, one needs to know the basics of the cooking process to design a simulation testing routine (if simulation tests are conducted) and to establish a schedule for the measurements. An examination of cooking practices will help the surveyor or researcher to assess how factors such as stove or pot type and certain operating conditions influence fuel consumption. Consequently, it may be possible to raise the overall fuel economy in an area by discouraging certain practices and encouraging others. A cooking practice survey will also reveal factors other than fuel efficiency (e.g. whether or not smoke serves any useful purpose) which should be taken into account, when designing or disseminating improved stoves.
The information which should be collected in a cooking practice survey includes:
1. the type (s) of fuel used for cocking throughout the year;
2. how fuel is obtained, its availability and price;
3. the types of food cooked and seasonal changes in diet;
4. the details of the cooking sequence for major meals;
5. details of the stove said pots;
6. other uses for the stove;
7. other cultural and physical conditions related to cooking.
In addition, it may be useful to consider the availability of materials and skills for constructing improved stoves during a cooking practice survey.
Questionnaires for cooking practice surveys have been included in a number of cookstove reports (deLepeleire, 1981; Dutt, 1981a; Joseph and Shanahan, 1980). One sample questionnaire is shown in Appendix 1. A questionnaire should be adapted to a particular area and pre-tested before being widely administered.
A few surveys of cooking practices and conditions have been carried out. For example, the food habits, cooking fuels, and other factors related to cookstoves have been studied in villages in four of the ten regions of Upper Volta (Ki-Zerbo, 1980).
Workers concerned with the development and introduction of improved cookstoves have also conducted limited studies of cooking practices including informal surveys in Senegal (Evans et al. 1980) and in a group of villages in South India (Geller, 1981a).
3.1 Fuel consumption
3.2 Energy consumption
3.3 Efficiency
3.4 Test parameter selection
The amount of fuel consumed during cooking is the simplest measurement that can be carried out. Fuel consumption should be measured by weight rather than by volume since it is difficult to measure accurately the volume of a nonuniform fuel such as wood. An individual fuel consumption measurement can be carried out during the preparation of one meal or one day's meals in a particular household. For this measurement, the fuel available to the cook is pre-weighed and the fuel remaining upon the completion of the meal (or the day's meals) is weighed in order to obtain the consumption during cooking.
Although field measurements of fuelwood consumption during cooking seem straightforward, problems can occur. For example, researchers working in two villages in Upper Volta had some difficulties carrying out a small set of daily wood consumption tests (Gern, et al. 1980). In these tests, some villagers did not understand or follow the instructions. One woman tried to minimize fuel consumption by heating up leftover food. In another house, some of the preweighed wood was lost. Thus, care must be taken during fuel consumption tests to see that additional fuel is not added to the preweighed pile and that the preweighed fuel is used only for cooking (Thomas et al. 1981). Furthermore, the standard food items should be prepared in the normal manner. In order to prevent procedural errors, it would be helpful if an individual from the survey team monitors each fuel consumption test.
In some developing nations, fuel type varies throughout the year. For example, in parts of the Sahel region, millet stalks are used instead of wood for several months of the year (Ki-Zerbo, 1980). Likewise, different food staples may be prepared throughout the year. In such areas, it may be necessary to measure fuel consumption separately for each food and/or fuel type in order to accurately estimate annual fuel consumption.
Some form of normalization can be useful for comparing cooking fuel consumption between different households or under different operating conditions. One simple normalization technique involves calculating specific fuel consumption (SFC) in terms of fuel consumption per family member. In this case, the units of SFC are kg/person/day or kg/person/meal. For this type of calculation, household size is sometimes calculated using weighting factors based on age and sex.1
1 For example weighting factors based on food intake or protein requirements, such as have been developed in League of Nations, 1932, and FAO/WHO, 1973.
SFC may also be defined in terms of the quantity of fuel consumed per unit of food cooked. Because the quantity of food cooked is taken into account explicitly, fuel consumption per unit of food may be a more accurate normalizing technique than fuel consumption per family member. However, this technique requires weighing food items as well as fuel. If such measurements are possible, then the food can be weighed either in its raw state prior to cooking or in the cooked state. 2
2 A step-by-step description of how to measure fuel consumption and specific fuel consumption was recently prepared by VITA, for cookstove researchers in West Africa (Downey, 1981).
There are a number of advantages to converting a measurement of fuel consumption into units of energy. First, conversion to energy units corrects for differences in fuelwood type and quality. Second, conversion to energy units is necessary in order to compare fuelwood consumption with other energy demands. In addition, a quantitative estimate of cooking energy demand is needed to evaluate the potential for alternative fuel sources and new cooking technologies.
Fuelwood consumption can be converted into units of energy consumption by multiplying by the calorific value of the fuel (MJ/kg). The calorific value of solid fuels is normally measured using a bomb calorimeter (Bialy, 1979b). Bomb calorimetry yields the higher heating value (HHV), also known as the gross calorific value. In this definition, the moisture produced and released during combustion is assumed to be in the liquid state. When solid fuels are considered, the HHV is usually given as the energy content (Earl, 1975). However, the moisture generated in a woodburning stove or an open fire usually remains in the gaseous state (Bialy, 1979b). The proper measure of the energy content in this case is the lower heating value (LHV), also known as the net calorific value. For air-dry wood, LHV is 6-8% less than HHV.1
1 Calorific value is also affected by whether the fuel burns at constant pressure or constant volume. However, the variation in calorific value between these two conditions is less than 1% for solid fuels (Bialy, 1979b). Therefore, this factor can be ignored during fuelwood surveys and the evaluation of fuelwood stoves.
A bomb calorimeter and other instruments needed for measuring calorific value cost about $1 000. Furthermore, these devices may be difficult to obtain in a developing country and some scientific training is required for their operations In addition, the bomb calorimeter and related instruments are somewhat delicate and they must be used in a laboratory. For these reasons, it may not be possible to directly measure fuel calorific value during a fuelwood survey.
As an alternative to bomb calorimetry, the calorific value of biomass fuels can be estimated with minimal error based on their moisture content and the known calorific value of dry fuels. Table III-1 shows the approximate calorific value of different biomass fuels on an oven-dry basis. The oven-dry calorific value is relatively constant for each type of fuel.2
2 There is a small difference between the calorific value of hard and soft wood; the value for soft wood is approximately 5% greater because of its higher tar and resin content (Arola, 1978). Also, the calorific value of peat can be somewhat higher than the value in Table III-1 if an older and deeper peat deposit is harvested.
In practice, the calorific value of fuel will differ from the values shown in Table III-1, mainly because of moisture content (Arola, 1978; Bialy 1979b). Moreover, this effect can be significant since moisture content varies over a wide range. Freshly cut wood can have a moisture content of 100% or more on a dry basis while air-dried wood typically has a moisture content of 10-20% on a dry basis (Bialy, 1979b).3 The actual moisture content of fuelwood depends on the drying time and conditions, the ambient temperature, relative humidity, and the characteristics of the fuel. In one rural area in South India, it was found that wood with an average moisture content of 10-20% on a dry basis is burned for cooking (Geller, forthcoming). On the other hand the use of relatively wet wood for cooking has been reported in Indonesia (Singer, 1961).
3 Moisture content on a dry basis (Mdb) is the ratio of the weight of the moisture in the wood to the weight of dry wood while the moisture content on a wet basis (Mwb) is the ratio of the weight of the moisture to the weight of the wet wood. Thus Mdb is always greater than or equal to Mwb and a moisture content of 100% or more is possible when calculating on a dry basis.
Table III-1 - Calorific Value (HHV) of Different Biomass Fuels on an Over-dry Basis
|
Fuel |
Calorific value (MJ/kg) |
|
Wood (a,b,c) |
20 |
|
Wood charcoal (a,b,c) |
31 |
|
Peat (b) |
21 |
|
Rice husks (a) |
15 |
|
Cow dung cakes (a) |
18 |
(a) Bomb calorimetry measurements by Geller at the Indian Institute of Science,
Bangalore, India, 1979-80.
(b) Bialy, 1979b.
(c) Earl, 1975
The actual calorific value of fuelwood Ew (HHV) can be estimated using Equation (1) if the moisture content of the fuel is known.
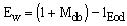(1)
where Mdb is the moisture content on a dry basis and Eod is the calorific value of the fuel when oven dried (see Table III-1).
The moisture content of wood is obtained by drying wood in an oven at approximately 100º C until its weight no longer changes. The quantity of moisture in the wood is determined by weighing the wood before and after drying. Thus, it is easier to estimate the calorific value of fuel through measuring its moisture content than by measuring calorific value directly. If this method of estimating calorific value is used, fuel samples should be stored in an air-tight container from the time of collection to the time of analysis.
For any fuel consumption test, it is possible to calculate specific energy consumption in the same manner as specific fuel consumption. Specific energy consumption normalizes for differences in fuel type as well as for differences in household size or the amount of food cooked. In summary, measuring fuel quantity and moisture content is a relatively simple yet accurate method for estimating energy consumption or specific energy consumption during fuelwood surveys.
Efficiency is a dimensionless quantity which is indicative of fuel economy. The analysis of cooking efficiency involves some normalizations in addition to those used for specific fuel or specific energy consumption. In particular, cooking efficiency takes into account the foods being cooked and the manner in which they are cooked. Thus, the determination of cooking efficiency facilitates comparison of fuel economy in situations where different foods are prepared or different cooking styles are used.
Cooking efficiency is normally defined as the fraction of the energy in the fuel consumed which has been usefully employed during cooking. The energy usefully employed during cooking can be defined as the energy used to heat the pot, the cooking medium, and the food to the cooking temperature(s) plus the energy absorbed by food as it cooks. This definition is reasonable for foods which are simmered in water (grains, legumes, boiled vegetables, etc.).
A general formulation of cocking efficiency as just described is given in Equation 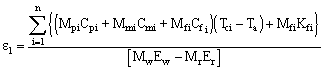(2).
(2)
The efficiency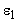 
applies to one complete cooking cycle. The summation in Equation (2) is over the different food items. For each item i, Mpi, Mmi, and Mfi are the masses and Cpi, Cmi, and Cfi, are the specific heats of the pots, cooking media, and foods respectively. Ta is the initial temperature of the pots, cocking medial and foods (normally the ambient temperature) and Tci is the cocking temperature of item i. Kfi is the energy required for the chemical reactions which take place during cooking a unit of item i. Mw and Ew are the mass and calorific value of the fuelwood consumed and Mr and Er are the weight and calorific value of any charcoal recovered upon completion of cooking.
The measurement and calculation of efficiency is simplest when water is the 0 cooking medium. In this case, the cooking temperature is approximately equal to 100 C at a pressure of one atmosphere. In order to calculate the efficiency using Equation (2), the following measurements or estimates have to be made:
(1) the weights of the pots, cooking water, foods, fuel consumed, and any charcoal reclaimed upon completion of cooking;(2) the temperatures of ambient air, cooking water at the start of cooking, and the contents of the pots during cooking;
(3) calorific values of the fuel and any recovered charcoal.
Thus, a scale and a thermometer are the only instruments needed to measure efficiency beyond those used for measuring or estimating calorific value.
The specific heats which may be needed to calculate efficiency include Cpi = 0.88 kJ/kgºC for clay pots, Cpi = 0,92 kJ/ kJ/kgºC for aluminium pots, and Cmi = 4.18 kJ/kgºC for water. The specific heats of selected foods are shown in Table III-2.
Table III-2 - Specific Heat of Selected Foods (a)
|
Food |
Moisture content |
Specific heat(b) |
|
Rice |
10.5 - 13.5 |
1.76 - 1.84 |
|
Flour |
12 - 13.5 |
1.80 - 1.88 |
|
Bread |
44 - 45 |
2.72 - 2.85 |
|
Lentils |
12 |
1.84 |
|
Meat |
39 - 90 |
2.01 - 3.89 |
|
Vegetable oil |
|
1.46 - 1.88 |
|
Milk |
87.5 |
3.85 |
|
Carrots |
86 - 90 |
3.81 - 3.93 |
|
onions |
80 - 90 |
3.60 - 3.89 |
|
Potatoes |
75 |
3.51 |
|
Apples |
75 - 85 |
3.72 - 4.02 |
(a) Table extracted from Charm, S.E., Fundamentals of Food Engineering, AVI Publishing, Inc., Westport, Connecticut, 1963.
(b) Average specific heat between 0º and 100º C.
Although the chemical reactions in food during cooking have been well-studied (Suzuki et al. 1976), these studies do not provide the energy absorbed by food during the cooking process (Kfi) in a convenient form. However, one set of experiments carried out in India deals directly with the question of the energy absorbed by food during the cooking process (Popali, 1979). Based on this study, the energy required for the chemical reactions in rice during cooking is estimated to be about 170 kJ/kg. Other cereals should have a similar cooking energy requirement. On the other hand, fresh vegetables will absorb a minimal amount of energy for chemical reactions during cooking since they contain 80% or more moisture. 1
1 The amount of energy taken up by food for chemical reactions during cooking is relatively small compared to the amount of energy used for the sensible heating of the food and the cooking medium. Therefore, overall efficiency is not sensitive to the assumptions regarding Kfi.
The measurement of cooking efficiency in the field is more difficult if cooking styles such as frying, baking, or grilling are encountered. For these cooking methods, there is a greater variation in cooking temperature compared to water-based cooking. Furthermore, cooking temperatures in excess of the boiling point of water are possible and the cooking temperature of the food may be different from that of the cooking medium. For example, fresh vegetables fried in very hot oil may not heat up above 100 ºC because of their high moisture content. For non-water-based cooking, thermocouples, thermistors, or bimetallic thermometers may be needed and more frequent temperature readings taken in order to estimate the cooking temperature. In such cases, the average temperature of the food during cooking can be used in the calculation of efficiency.
Some cooking techniques involve water evaporation as an essential part of the cooking process. This includes stewing or steaming. For these cooking styles, it is reasonable to count the energy required for evaporating water as part of the energy usefully employed during cooking. Cooking efficiency in this case is given by Equation 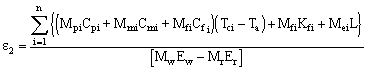(3).
(3)
In Equation (3), Mei is the mass of water evaporated from pot i. Mei = 0 if water evaporation is not needed for cooking in a particular pot. L, the latent heat of evaporation of water, is 2260 kJ/kg. The amount of water evaporation can be easily measured by weighing the full pot before and after cooking.
Water evaporation is also an integral part of cooking breads and other baked or grilled foods. Therefore, the energy used for water evaporation should be included as energy usefully employed for these foods. Furthermore, the energy required for water evaporation can be significant with these foods. For example, Geller found that when chappatis (flat breads) are cooked on an open pan in South India, the energy used to evaporate water in the dough is about 2-5 times the energy used to raise the temperature of the dough. When cooking food items such as bread, the amount of water evaporation is also measured by weighing the item before and after cooking.
A number of indicators of cooking fuel economy have been proposed in this section. These include:
1. fuel consumption per meal or day;
2. specific fuel consumption in terms of fuel weight per person;
3. specific fuel consumption in terms of fuel weight per unit of food;
4. energy consumption per meal or day;
5. specific energy consumption;
6. efficiency.
Of course, the researcher or surveyor Must select one or more of these parameters for use in the fuelwood survey. This selection could be based on practical concerns such as equipment availability, complexity, time, and cooperation from the population being surveyed. Complexity, equipment requirements, and the degree of interference in the cooking process generally increase when proceeding down the list above.
It is also possible to select a test parameter according to the analytical precision desired. In this case, the objective is to choose a parameter which shows the least amount of variation between similar tests. The method for calculating the degree of variation within a series of tests is explained in section VI. In general, the degree of variation is expected to decrease as test sophistication increases. This result is due to the increasing application of normalization techniques as the test parameter proceeds from fuel consumption to efficiency.
In order to check the above hypothesis and to select the test parameter with the least variation during an actual fuelwood survey, a preliminary series of tests would have to be conducted. During the preliminary tests, all test types are analysed for degree of variation, Based on these results, a particular test can be selected for the survey as a whole.
In reality, it is likely that some compromise between test complexity and the degree of variation will be needed. If this is the case, specific fuel consumption in terms of fuel and food weight seems to be a reasonable "compromise" testing parameter. The measurement of this parameter is relatively simple and involves only minimal interference in the cooking process (weighing fuel and raw food). The measurement or estimation of fuel calorific value-is not required. Furthermore, the sacrifice in precision with this parameter may be small. For example, field tests in a cluster of villages in India showed similar degrees of variation as well as a high correlation between specific fuel consumption (in terms of fuel weight per unit of food) and efficiency (Geller, forthcoming). Therefore, specific fuel consumption should suffice as a comparative measure of fuel economy in many surveys. This is more likely to be the case if the foods and fuels are reasonably consistent from test to test within the survey.
Fuel consumption and cooking efficiency tests in the field require the surveyor or researcher to interfere with cooking to some extent. While the level of inconvenience should be minor, it may be difficult to conduct field tests in some locations, Furthermore, fuel consumption and efficiency tests during cooking are highly task and site specific. This makes it difficult to compare fuel economy between different regions.
Because of these problems, the performance of fuelwood cookstoves is often measured using laboratory-based simulation tests. Simulation tests usually involve only the heating and boiling of water. Therefore, they are much simpler than cooking tests. Critical parameters such as fuel and pot types are kept constant during a series of simulation tests unless the effect that one of these parameters has on efficiency is being studied. It may be useful to include simulation tests in a fuelwood survey if cooking tests are not possible or if detailed information regarding cooking fuel economy under controlled conditions is desired.
The term "heat utilized" has been proposed as the figure of merit in simulation tests (Joseph and Shanahan, 1980). Heat utilized is generally the fraction of the energy in the fuel consumed during the simulation test which contributes to either water heating or water heating and water evaporation. Thus, the heat utilized during a simulation test is similar to the definition of cooking efficiency during field tests. Equations (2) and (3) can be used to calculate the heat utilized during simulation tests if the terms involving food are ignored.
The question also arises whether or not to consider evaporative energy as heat utilized in simulation tests. Including water evaporation as heat utilized takes into account all the energy absorbed by the water. However, this method of calculating heat utilized could be misleading when applied to situations where water evaporation is not essential for cooking. Therefore, for simulation tests, it is preferable to measure and report heat utilized using both methods.
A number of simulation test procedures have been used in the past (Morgan et al., 1979). These include:
1. a fixed quantity of fuel is burned and a specific amount of water is repeatedly heated to a certain temperature;2. a fixed quantity of fuel is burned and the amounts of water heating and evaporation are measured;
3. a fixed quantity of water is heated to boiling and the amount of fuel consumed is measured;
4. a fixed quantity of water is heated to a boil and is then kept simmering for a certain period of time while the amount of fuel consumed is measured;
5. a fixed quantity of water is completely evaporated and then the fuel consumed is measured.
Some of these simulation test procedures may not be representative of actual cooking practices. Furthermore, it has been shown that the optimal heat output level and the efficiency of a stove varies significantly for different operations such as heating water or simmering water (Dutt, 1978), A stove which is relatively efficient for one function may give a poor efficiency for another. Therefore, a simulation test procedure which deviates significantly from the actual cooking routine in a region may not give meaningful results.
Some cookstove researchers are now using simulation test procedures which are based to a greater extent on the way people cook (Joseph and Shanahan, 1980). For example, a simulation test tailored to the cooking routine in a particular region of South India includes periods of water heating, simmering, and the rotating of pots between stove openings (Geller, 1981b). Simulation testing using a procedure reflecting local cooking patterns is recommended even though it may limit the potential to compare test results between different studies.1
1 For a detailed explanation of how to conduct simulation tests in this manner, see VITA's stove testing manual. Downey, 1981.
The heat utilized during simulation testing can be much higher than the efficiency realized in the field. Under optimal conditions, heat utilized values of close to 30% have been obtained in simulation tests on an open fire (Krishna Prasad, 1980).2 This is 2-6 times higher than the efficiency generally reported or assumed for open fire cooking (Morgan et al., 1979; Krishna Prasad, 1980). Even when a simulation test procedure reflects local cooking practices, differences in fuel economy between simulation and cooking tests can result. Geller, for example, measured heat utilized values which were 25-30% higher than the actual cooking efficiencies with traditional stoves in South India (Geller, 1981 and forthcoming). Thus, simulation tests should be viewed as an indicator of relative fuel economy rather than as an indicator of absolute performance.3
2 In these tests, the energy absorbed for water evaporation is included as heat utilized.
3 This is analagous to the situation with automobile fuel economy and the specific gel consumption tests conducted by the U.S. Environmental Protection Agency (EPA). Fuel economy based on EPA's simulation tests are generally higher than the "on-the-road" values (Gray and vanMippel, 1981).
5.1 Test type in relation to testing objective
5.2 Variability between tests
5.3 Comparison of the relative fuel usage of two stoves
The selection of a testing methodology depends in part on the overall objective of the tests. Tests may be conducted for one or more of the following reasons:
(a) to measure fuel or energy consumption during cooking;
(b) to compare the relative fuel usage of two (or more) stoves;
(c) to check how factors such as pot or fuel type affect fuel consumption;
(d) to understand the distribution of heat in a stove in order to minimize fuel use;
(e) to optimize the dimensions of a stove in order to minimize fuel use.
Table III-3 shows the test procedures which can be used with the different testing objectives. Testing procedures fall into two categories - those carried out in a laboratory and those conducted in the field. Furthermore, they can involve one, a few, or many stoves and either cooking or simulation tests. Objectives (a), (b) and (c) are of the greatest interest to fuelwood surveyors, forestry planners and extension agents. Cookstove researchers, however, may be interested in all of the objectives.
Table III-3 Relationship between Objective and Testing Procedure (*)
|
Number of stoves |
Laboratory tests |
Laboratory cooking tests |
Field cooking tests |
|
1 |
(c) (d) (e) |
- |
(c) |
|
2-5 |
(b) (c) |
(b) |
(c) |
|
6 or more |
- |
- |
(a) (b) |
* Letters in parentheses refer to the testing objective as listed in the text above.
As shown in Table III-3, objective (a) requires field data from many households. Objective (b), on the other hand, can be satisfied using either laboratory or field measurements depending on the circumstances. A researcher developing efficient stoves would first compare different stoves using simulation tests. Once a promising design is developed in the laboratory, actual cooking tests should be conducted in order to verify the simulation test results. A testing procedure for comparing the relative fuel usage between stoves is described later in this section.
Tests of how critical variables affect fuel economy (objective (c)), can be carried out either in the field or in the laboratory. As described in section 6, the data collected in field surveys of fuelwood consumption can be statistically analysed to determine whether certain variables are associated with higher than average or lower than-average efficiencies. It is also possible to consciously study how factors such as pot or fuel type influence efficiency through comparative testing in the laboratory.
Only stove researchers would be concerned with objectives (d) and (e) since many measurements are required in both cases. Furthermore, tests to satisfy objectives (d) and (e) involve complicated studies of the combustion and heat transfer phenomena in fuelwood stoves. These studies are only realistic in the laboratory.
Fuel consumption and efficiency can vary significantly from meal to meal on woodburning stoves in a particular area. This is due to variations in stoves, fuels, the foods being cooked, and the operating methods of the cook. For example, a three-fold variation in cooking efficiency was observed between homes in a group of South Indian villages (Geller, forthcoming). The degree of variation in fuel economy between meals can result in a high level of statistical uncertainty in a series of cookstove performance tests. However, the testing methodology can be designed to minimize the variation and/or the range of uncertainty for a series of tests conducted under similar conditions.
The selection of the test parameter is one factor which can be used to limit the variation in field tests. As mentioned in section 3, the surveyor or researcher has the choice of measuring fuel Consumption, specific fuel consumption, energy consumption, or efficiency. The degree of variation during a series of similar tests should in general decrease as the test parameter proceeds from fuel consumption to efficiency. This phenomenon is demonstrated quantitatively in an example in section 6.
It is also possible to use the field testing procedure to limit the statistical variation between similar tests. One testing procedure involves selecting a large number of households with only one measurement or just a few measurements in each household. This is known as a cross-section study. It characterizes the variation in fuel use or efficiency among households without giving any indication of the variation within a household. A second method, known as a time series study, involves a large number of measurements over a period of time in an individual household. Of course, conducting some tests in a number of households for example, six tests in each of eight households) will provide information on the variation within as well as across houses. In order to minimize the range of uncertainty associated with a test parameter, the tests should be targeted where the degree of variation is greatest. For example, if there is greater variation between households rather than within households, then a larger number of households should be sampled with fewer tests per house.
The evaluation of the relative fuel usage between two stoves is an important element in Stove development programmes. This is also a commonplace testing requirement. Measurements of this type should be done by the stove designer or surveyor prior to attempting to introduce a new stove. In addition, measurements conducted 6 to 12 months after stove introduction are desirable in order to assess the longer-term impact on fuel use. When comparing the performance of two stoves, it is especially important to reduce the effects of variability due to fuel type, moisture content, food cooked and cooking style. One procedure for comparison testing was first developed in Kaya, Upper Volta (Dutt, 1981b).1
1 The Kaya procedure is presented as a method to compare two different stoves. It can also be used to compare fuel economy under two different operating conditions (e.g. clay vs. aluminium pots).
In the Kaya procedure, each participating household is given both of the stoves being compared. The cook(s) must agree to alternate their cooking between the two stoves. Measurements of one of the test parameters described in section 3 are repeated each meal or day. A minimum of 5 households and 6 consecutive days of cooking tests per house are essential. The Sample size depends on the level of accuracy sought and is discussed in Appendix III-4.
Alternate cooking tests should follow a certain schedule in order to avoid cooking the same meal on the same stove each day. For locations such as Kaya where two meals are cooked per day, the schedule presented in Table III-4 can be used.
Table III-4 - Kaya Procedure
|
Day 1 |
midday meal: |
Stove A |
|
evening meal: |
Stove B |
|
|
Day 2 |
midday meal: |
Stove B |
|
evening meal: |
Stove A |
|
|
Day 3 |
midday meal: |
Stove A |
|
evening meal: |
Stove B, etc. |
The test should be run for an even number of days so that each stove is used the same number of times for a particular meal. A data sheet for the Kaya procedure is shown in Appendix III-2.
6.1 Characterizing a series of tests
6.2 Data analysis in comparative tests
A series of tests yields many measurements of the same parameter(s). These measurements may be described in terms of the arithmetic mean. If Xi is the ith of n measurements of the quantity X, the arithmetic mean is given by
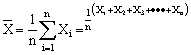(4)
The arithmetic mean or average does not describe the variability in the measurements. The variability is given by the standard deviation S where
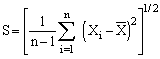(5)
The standard deviation divided by the mean yields a parameter known as the coefficient of variation (COV). COV is a normalized measure of variability which is independent of the units of the quantity being measured.
Table III-5 shows an example of field tests in 13 households (Geller, 1981a). This is a relatively small sample and, consequently, the results are only indicative of the conditions in the population as a whole., Nonetheless, this example can be used to illustrate the analysis of test data. For the 13 tests shown in Table III-5, the average fuelwood consumption is 3.61 kg per household and the average efficiency is 6.0%. The mean, standard deviation, and COV for various test parameters are shown in Table III-6.
It is seen that the COV decreases from 59% for fuel consumption to 36% for efficiency. However, the COV for specific fuel consumption (in terms of food quantity) is only slightly greater than the COV for efficiency. This example supports the conclusions in previous sections that:
1. the coefficient of variation decreases as the complexity of the test parameter increases;2. from the viewpoint of reducing variability, specific fuel consumption (in terms of fuel consumed per unit of food cooked) is nearly as good a parameter as efficiency in field tests.
Table III-5 - Field Tests in 13 Households in the Ungra Village Area of South India (Geller, 1981a)
|
Household Size |
Predominant pot type (a) |
Quantity of food cooked (kg/meal) |
Firewood consumed (kg/meal) |
Efficiency (%) |
|
3 |
C |
1.55 |
2.90 |
3.2 |
|
4 |
Al |
2.29 |
1.58 |
7.7 |
|
4 |
Al |
1.73 |
3.40 |
3.9 |
|
5 |
Al |
2.35 |
2.15 |
7.4 |
|
6 |
Al |
2.47 |
3.51 |
5.8 |
|
6 |
C |
2.87 |
4.15 |
3.8 |
|
7 |
C |
2.39 |
2.16 |
6.7 |
|
8 |
Al |
3.02 |
2.06 |
9.2 |
|
8 |
Al |
4.42 |
2.35 |
8.4 |
|
9 |
Al |
4.55 |
3.99 |
7.0 |
|
9 |
C |
5.07 |
8.12 |
3.4 |
|
10 |
C |
4.82 |
8.03 |
3.6 |
|
11 |
Al |
2.96 |
2.51 |
8.3 |
(a) C - Clay; Al - Aluminium
Table III-6 - Variability in the Different Test Parameters from 13 Field Tests in South India (a)
|
Parameter |
Mean |
Standard deviation(S) |
Coefficient of variation |
|
Fuel consumption (kg/meal) |
3.61 |
2.13 |
.59 |
|
Specific fuel consumption |
0.550 |
0.264 |
.48 |
|
Specific fuel consumption |
1.208 |
0.465 |
.38 |
|
Efficiency (%) |
6.03 |
2.18 |
.36 |
(a) Table based on the work of Geller at the Indian Institute of Science, Bangalore, India. See Table III-5 for the primary data.
The comparison of fuel consumption or efficiency between two different stoves or two different operating conditions is a common testing objective. For example, in improved stove projects, there is a need to compare the fuel economy of a new stove with a traditional stove (or an open fire). A procedure for conducting comparative tests was presented in the previous section. Here, we give some techniques for determining whether the test parameter is significantly different for the different stoves or operating conditions and, if so, the uncertainty in the difference and the level of significance on a statistical basis.
The simplest way to compare two sets of data is to compare the means from each set. However, there will be some uncertainty in the difference of the two means due to the distribution of the measurements. The level of uncertainty can be estimated using the standard deviation as shown in the following example.
Table III-7 shows the results from a set of tests comparing fuel consumption during cooking for an open fire and a new cookstove designed by a Peace Corps volunteer in Kaya, Upper Volta (Hooper, 1980). The tests were carried out by Peace Corps volunteers following the procedure described in the previous section (Schroeder and Lavell, 1981). In this set of tests, fuel consumption with the Kaya stove was compared with the open fire in six households with 9 - 13 tests conducted per house. Table III-7 shows that the average fuel savings with the stove is 1.01 kg per meal or 28%. A crude estimate of the uncertainty in this value can be obtained by calculating the standard deviation in the average savings per household.1 The standard deviation for these values is 0.43 kg per meal or 12%. Thus, the estimate of the savings with the Kaya stove may be stated as 1.01 ± 0.43 kg per meal (28% ± 12%).
1 This estimate is crude because we have not taken into account the fact that there were several tests done with each-stove in each household.
Table III-7 - Fuel use per Meal in open fires and Kaya Stoves
|
Household |
Household |
Average wood use per meal (kg) (a) |
Fuel savings with the Kaya stove relative to the open fire | ||
|
|
|
open fire |
Kaya stove |
(kg/meal) |
(%) |
|
1 |
12 |
3.72(5) |
3.00 (4) |
0.72 |
19 |
|
2 |
6 |
3.69(7) |
2.84 (5) |
0.85 |
23 |
|
3 |
8 |
2.58(6) |
1.88 (6) |
0.70 |
27 |
|
4 |
14 |
4.45(4) |
3.05 (6) |
1.40 |
31 |
|
5 |
6 |
3.82(6) |
2.13 (7) |
1.69 |
44 |
|
6 |
10 |
3.10(4) |
2.42 (6) |
0.68 |
22 |
|
Average |
|
3.56(32) |
2.55 (34) |
1.01 |
28 |
(a) The numbers in parentheses-refer to the number of meals over which the fuel consumption has been averaged.
The "t-test" is another method for statistically comparing sets of measurements for two stoves or two operating conditions. In order to perform the t-test, the mean and standard deviation for each group of tests must be calculated. Then, the t value can be computed from Equation (6).
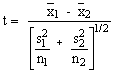
where the subscripts 1 and 2 denote each stove or operating condition and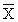 
, S, and n are the mean, standard deviation, and the number of tests respectively for each situation.
The computed t value is compared to values in a t-table to determine if the mean from one group is significantly greater than the mean from the other. An abridged t-table is shown in Appendix III-3. The values in the table are listed as a function of the "degrees of freedom" and level of significance. Degrees of freedom is simply the number of test measurements minus the number of parameters which have been estimated based on the measurements. The level of significance is the percentage chance that the result indicated by the t-test is not true. Therefore, the statistical difference between the means from the two groups increases as the indicated level of significance decreases.
The t-table is used by comparing the calculated t-value to the numbers in the table at the appropriate degrees of freedom. It can be said that the mean from one group of tests is greater than the mean from the other at a certain level of significance if the computed t-value is greater than the number in the table at that level.
The application of the t-test may be illustrated using the field test data from South India (Table III-5). The measurements in this table can be divided into two groups according to the predominant pot type, clay or aluminium. Table III-8 shows the mean and the standard deviation in the specific fuel consumption for each group. Using Equation (6), the t-value for this example is 2.20. Furthermore, there are 11 degrees of freedom in the example since 13 measurements were made and two parameters (the means for each group) were estimated, Based on the entries in the t-table at 11 degrees of freedom, the t-value is greater than the number in the table at a level of significance of 2.5% but less than the number at 1.0%1
1 The significance level can be improved by increasing the sample size (see Appendix III-4).
This series of tests suggests that households using aluminium pots have a high probability of saving fuel compared to households using clay pots. This does not, of course, prove that the use of aluminium pots is more fuel efficient than the use of clay pots. Households using aluminium pots may have other characteristics that distinguish them from households cooking with clay pots. For example, pot type might correlate with a difference in socio-economic level or diet and one of these latter factors might cause the difference in fuel usage. For a more definitive assessment of the influence of cooking pot type (or some other variable) on fuel use, we recommend alternating pot types in the same household following the Kaya procedure.
Table III-8 - Difference in Specific Fuel Consumption between Households Using either Clay or Aluminium pots in South India
|
Predominant pot type |
Number of Households |
Specific Fuel Consumption |
|
|
|
Mean |
Standard deviation |
|
|
Clay |
5 |
1.50 |
0.36 |
|
Aluminium |
8 |
0.99 |
0.47 |
Appendix III-1 - Cooking survey questionnaire
Appendix III-2 - Kaya procedure data sheet
Appendix III-3 - T-Table
Appendix III-4 - Choosing appropriate sample sizes
The objectives of this report are to present:
1. the reasons for testing the fuel economy of cookstoves;
2. the methods for measuring stove performance in the field and in the laboratory;
3. the procedures for conducting a series of tests;
4. the techniques for interpreting test results.
There are a variety of parameters which can be measured during cooking. These include fuel consumption, specific fuel consumption, energy consumption, and efficiency. Energy consumption can be estimated with minimal error by measuring the quantity and the moisture content of the fuel. Efficiency requires more detailed measurements. Furthermore, specific fuel consumption is simple to measure yet It appears to be nearly as accurate as the more sophisticated testing parameters. Therefore, it is recommended when field testing.
Simulated cooking tests are useful for comparing the efficiency of different stoves or different operating conditions. These tests are conducted in the laboratory and involve the measurement of "percentage heat utilized". When performing simulation tests, we recommend developing a water heating and simmering routine based on the local cocking practices, Simulation tests should be used to gauge relative fuel economy rather than the absolute efficiency which can be expected during cooking.
There will be some variability in the measurement of fuel consumption, specific fuel consumption, or efficiency during a series of tests. However, a series of tests can be structured to limit this variability. A carefully designed testing procedure is also useful for comparing the relative fuel economy of two stoves or two different operating conditions.
In order to draw conclusions from a series of fuel economy tests, it is useful to calculate certain statistical functions. These include the mean, standard deviation, coefficient of variation, and t-value. These parameters can be used to estimate the level of uncertainty of the significance of a test result.
* This survey was designed by Andre Longmire (Peace Corps, Mali)
1) Background
|
Name of village: |
||||
|
Household size:_______ |
Adults __________ |
Children (under 16) __________ |
||
|
Number of people for whom food is cooked (average): |
__________:Adults |
|||
|
|
Children (under 16) __________ |
|||
|
Who does the cooking |
#1 __________ |
How often: |
__________ |
|
|
#2 __________ |
|
__________ |
||
|
#3 __________ |
__________ |
|||
|
Type of meal usually cooked: |
||||
|
Morning |
________________________________________ |
Time __________ |
||
|
Afternoon |
________________________________________ |
Time __________ |
||
|
Evening |
________________________________________ |
Time __________ |
||
|
Cooking process for each item cooked: |
||||
|
1. |
||||
|
2. |
||||
|
3. |
||||
|
4. |
||||
2) Cooking
|
Are there customs centered around use or placement of the stove or place of cooking? Yes/No. If Yes, describe________________________________________________________________ | ||
|
Do they cook inside or outside?__________________________________________________ | ||
|
State no. of months in each: |
inside__________________ |
outside:__________________ |
|
In the cold season do they cook inside to keep warm? Yes/No | ||
|
If they cook mostly inside now and if an indoor stove released smoke outside, would they mind cooking inside most of the time? Yes/No | ||
|
If the stove is inside, does it have a chimney? Yes/No | ||
|
If stove is inside without a chimney, does the smoke serve any useful purpose? | ||
|
Yes/No; If yes, what? __________________________________________________________ | ||
|
Comments: __________________________________________________________________ | ||
3) Stoves - Describe stoves below, listing them by frequency of use.
|
Stove No. 1 (used most often) |
|
Type: Three stone/2 hole without flue/2 hole with flue |
|
Age of Stove: |
|
Location: inside/outside/under a shelter/other _______________________________________ |
|
Months of use ______________________________ |
|
Frequency of use: daily/3 to 5 times a week/1 or 2 times a week/other ____________________ |
|
Which meals are prepared on it: __________________________________________________ |
|
Types of pots most frequently used (metal, clay, etc.) __________________________________ |
|
Which pot sizes fit this stove best: ________________________________________________ |
|
Who built the stove? ___________________________________________________________ |
|
How much would it cost now? ____________________________________________________ |
|
What are the materials used in its construction? _____________________________________ |
|
How long would it last? _________________________________________________________ |
|
How is it maintained? __________________________________________________________ |
|
Stove No. 2 (used next most frequently) |
|
(Repeat as in stove No. 1.) |
|
What other functions do the stove(s) serve besides cooking? ___________________________ |
4) Fuel
|
What is the most common fuel source: ________; other ________; |
______________ |
||||||
|
When wood is used as fuel, what is the approximate length ______; |
diameter_____ |
||||||
|
Type of wood preferred |
#1 __________ |
||||||
|
#2 __________ |
|||||||
|
#3 __________ |
|||||||
|
Reasons: ___________________________________________________________________ |
|||||||
|
Does the household often buy wood? Yes/No |
|||||||
|
If Yes, |
|||||||
|
|
Is wood bought all year? Yes/No (specify) ________________________________________ |
||||||
|
What is the range of prices paid? ________ |
to _______________ |
per ______________ |
|||||
|
Dry season to per ____________________ |
to _______________ |
per ______________ |
|||||
|
Rainy season to per __________________ |
to _______________ |
per ______________ |
|||||
|
Other times to per ____________________ |
to _______________ |
per ______________ |
|||||
|
How much does it cost now per week? |
|||||||
|
_____________ |
at a price of |
_____________ |
|||||
|
If No, (i.e., family rarely buys wood) |
|||||||
|
Do they use wood from their own trees? Yes/No |
|||||||
|
Do they gather wood? Yes/No |
|||||||
|
If they gather wood, who gathers? (specify relationship to head of household)_______________ |
|||||||
|
How often?__________________________________________________________________ |
|||||||
|
Roughly how much is gathered during each trip?______________________________________ |
|||||||
|
How long does it take for each trip? _______________________________________________ |
|||||||
|
Do they store their wood dry?____________________________________________________ |
|||||||
|
If yes, how long?______________________________________________________________ |
|||||||
5) Local materials and technology (a)
(a)This part of questionnaire need only be asked of a few families in any village.
|
Are there locally made stoves, ovens or other cooking devices? Yes/No |
|
If yes, describe how they are used and how often; how they are made and by whom __________ |
|
Are there masons in the area? ___________________________________________________ |
|
Do the people use bricks, cement, etc. for masonry construction? ________________________ |
|
What do the local people use for mortar for their granaries and houses? |
|
Traditionally what is the best mortar? Describe ______________________________________ |
|
Is there someone in the village who makes pottery? Yes/No If yes, describe the type of pottery; how it is made; what materials are used. |
|
Are there stone-cutters in the area? Yes/No |
|
What is the local process for drying vegetables and fish? ______________________________ |
|
Stove A: __________ |
Pot sizes: |
Pot type(s): | ||||||
|
Stove B: __________ |
Pot sizes: |
Pot type(s): | ||||||
|
Principal cook (P.C.)____________________(relationship to head of household) | ||||||||
|
|
|
|
|
Weight of wood |
|
|
| |
|
Date of cooking |
Meals cooked (M/E) (1) |
Cook (2) |
Stove used (A/B) (3) |
Before cooking (kg) |
After cooking (kg) |
Number people eating meal |
Leftovers saved for later meal |
Comments on type of wood |
|
|
|
|
A |
|
|
|
|
|
|
|
|
|
B |
|
|
|
|
|
|
|
|
|
B |
|
|
|
|
|
|
|
|
|
A |
|
|
|
|
|
|
|
|
|
A |
|
|
|
|
|
|
|
|
|
B |
|
|
|
|
|
|
|
|
|
B |
|
|
|
|
|
|
|
|
|
A |
|
|
|
|
|
(1) M: mid-day meal; E: evening meal; specify M or E
(2) Enter P.C. if weal was cooked by principal cook. Otherwise enter relationship of cook to head.
(3) If a different stove is used than indicated on schedule, please note it.
(4) Specify if wood is wet or dry, large pieces or twigs. Enter additional comments on reverse along with relevant data and time.
|
Degrees of freedom |
Level of significance(%) (a) |
|
|||
|
10 |
5 |
2.5 |
1 |
0.5 |
|
|
1 |
3.08 |
6.31 |
12.7 |
31.8 |
63.7 |
|
2 |
1.89 |
2.92 |
4.30 |
6.96 |
9.92 |
|
3 |
1.64 |
2.35 |
3.18 |
4.54 |
5.84 |
|
4 |
1.53 |
2.13 |
2.78 |
3.75 |
4.60 |
|
5 |
1.48 |
2.01 |
2.57 |
3.36 |
4.03 |
|
6 |
1.44 |
1.94 |
2.45 |
3.14 |
3.71 |
|
7 |
1.42 |
1.90 |
2.36 |
3.00 |
3.50 |
|
8 |
1.40 |
1.86 |
2.31 |
2.90 |
3.36 |
|
9 |
1.38 |
1.83 |
2.26 |
2.82 |
3.25 |
|
10 |
1.37 |
1.81 |
2.23 |
2.76 |
3.17 |
|
11 |
1.36 |
1.80 |
2.20 |
2.72 |
3.11 |
|
12 |
1.36 |
1.78 |
2.18 |
2.68 |
3.06 |
|
13 |
1.35 |
1.77 |
2.16 |
2.65 |
3.01 |
|
14 |
1.34 |
1.76 |
2.14 |
2.62 |
2.98 |
|
15 |
1.34 |
1.75 |
2.13 |
2.60 |
2.95 |
|
16 |
1.34 |
1.75 |
2.12 |
2.58 |
2.92 |
|
17 |
1.33 |
1.74 |
2.11 |
2.57 |
2.90 |
|
18 |
1.33 |
1.73 |
2.10 |
2.55 |
2.88 |
|
19 |
1.33 |
1.73 |
2.09 |
2.54 |
2.86 |
|
20 |
1.32 |
1.72 |
2.09 |
2.53 |
2.84 |
|
21 |
1.32 |
1.72 |
2.08 |
2.52 |
2.83 |
|
22 |
1.32 |
1.72 |
2.07 |
2.51 |
2.82 |
|
23 |
1.32 |
1.71 |
2.07 |
2.50 |
2.81 |
|
24 |
1.32 |
1.71 |
2.06 |
2.49 |
2.80 |
|
25 |
1.32 |
1.71 |
2.06 |
2.48 |
2.79 |
|
26 |
1.32 |
1.70 |
2.06 |
2.48 |
2.78 |
|
27 |
1.31 |
1.70 |
2.05 |
2.47 |
2.77 |
|
28 |
1.31 |
1.70 |
2.05 |
2.47 |
2.76 |
|
29 |
1.31 |
1.70 |
2.04 |
2.46 |
2.76 |
|
30 |
1.31 |
1.70 |
2.04 |
2.46 |
2.75 |
|
00 |
1.28 |
1.64 |
1.96 |
2.33 |
2.58 |
(a)This is the one-sided level of significance which is applied when testing whether the mean from one population is greater than the mean from another.
The level of significance with which the means of two samples of data can be distinguished depends on the number of measurements (sample size), the standard deviation of the measurements, and the difference between the sample means. If there are n measurements in each sample, the standard deviation is a fraction "a" of the population mean, and the difference between the sample means is a fraction "d" of the population mean, i.e., s = ax and x1 - x2 = dx, then, for a significance level of µ,
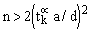
where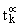 
is the t value corresponding to a significance level of µ and k degrees of freedom. In our example, k = 2n-2. Both sides of the inequality (A.4.1) depend on n. Fortunately, 
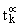is only weakly dependent on k and hence on n. For n greater than about 10, t = 2.0 for a 2.5% level of significance and t = 2.5 for a 1.0% level of significance (see Appendix III-3).
We have seen in an earlier stove test that a = 0.4. Thus, using A.4.1, for a 2.5% level of significance, n needs to be at least 2 (2.0)2 0.16/d2=1.28/d2. For a 1.0% level of significance, n should be greater than 2.0/d2. (See the accompanying table for minimum sample sizes at specific values of d and x). For example, given a 20% difference in mean (d=0.2) and a 2.5% level of significance, n should be greater than 32. Of course, a researcher will not know beforehand what the value of d is going to be. Nonetheless, a sample size can be selected based on the anticipated difference, in means and the level of significance desired.
Table III-9 - Minimum Sample Size for Various Percent Differences in Means and Levels of significance
|
Percent difference in means (100 d%) |
Level of significance (µ) | |
|
|
2.5% |
1.0% |
|
10 |
128 |
200 |
|
20 |
32 |
50 |
|
30 |
14 |
22 |
|
40 |
8 |
13 |
|
50 |
5 |
8 |
)