SECTION 5. MORTALITIES

5.1 Introduction
5.2 Estimation of total mortality
5.3 Fishing mortality
5.4 Natural mortality and growth characters
5.5 Special graphical methods
5.6 Exercises

5.1 Introduction

When studying mortalities we are concerned with rates of change, and it is generally most convenient to deal with instantaneous rates of change (see section 1); that is, the rate at which the numbers in the population are decreasing can be written as

where Z is defined as the instantaneous total mortality coefficient. From this equation the number N_t alive at any time will be given by (see section 2.4.1, exercise No. 18).

N_t = N₀e-^Zt

where N₀ = number alive at time = 0.

Often we are concerned with considering separately two causes of removal from the population - natural deaths (disease, predation, etc.) and fish caught; then we can write

where M = natural mortality coefficient

where F = fishing mortality coefficient

then in a very short time interval dt, the deaths due to fishing will be equal to FNdt, natural deaths MNdt, and total deaths ZNdt, therefore

F + M = Z

i.e. instantaneous mortality coefficients are additive.

5.2 Estimation of total mortality

Before estimating the fishing and natural mortalities separately, it is convenient to estimate the total mortality. This is readily done if we know the abundances, say N₀, N₁ of any group of fish at two known times, for then the fraction surviving is 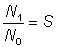, and the total mortality coefficient is given by

.................... (5.1)

or

This has the obvious special case where t = one year. The same formulae also hold when the true abundances are not known, but indices of them, e.g. catches per unit effort, are available, i.e. number n₀, n₁ proportional to N₀, N₁ then

Often abundances, or indices of abundance, will not be known at any particular exact time, but only as averages over finite periods of time, e.g. a year. In terms of the abundance at the beginning of the period, N, and the total mortality coefficient, Z, the average abundance during the period, duration T, may be denoted by N; the numbers alive at time t are Ne^-Zt so that

If we denote by appropriate suffixes the values of numbers and mortalities during periods 0 and 1, of duration T, starting a year apart we have

and also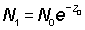

Taking the ratio of the average abundances, we have

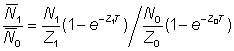
............... (5.2)

If Z₀ = Z₁ = Z, then equation (5.2) reduces to

i.e. the ratio of the mean abundance can be used in the same manner as the ratio of instantaneous abundances in equation (5.1).

The simplification can also be made if Z₀ T and Z₁ T are both small, even if not equal, for then 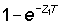is nearly equal to Z₁T and similarly for 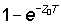. The last two factors in equation (5.2) then cancel out, and equation (5.2) becomes 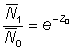. The ratio of the mean abundances during two periods therefore provides a good estimate of the survival during the year if either the mortality is constant, or the change in numbers during both periods is small. Even if these conditions are not satisfied, the ratio may still provide a fair first approximation, subject to corrections which may be deduced from equation (5.2).

The formulae above will usually be applied to the abundances of individual year-classes and enable estimates to be made of the mortalities between any pair of successive years and for any fully recruited year-class. These estimates are likely to be quite numerous, and the question then arises as to how the "best" combined estimate of mortality can be obtained. A single combined estimate is only valid if there are no systematic differences in mortality, either with age or time, over the period considered; these may be most easily detected from an array such as Table 5.1, either by inspection of the means of rows and columns, or more rigorously by a statistical test, such as the analysis of variance.

The entries in such a table should always be in the form of instantaneous coefficients, not percentage mortalities, as it is easy to show that the latter are biased, being underestimated by an average amount depending on the variance of the data, and that this bias may be quite appreciable when taking the mean of several estimates. This bias is due to the numerator in equation (5.1) being not known exactly; the overestimate of the survival when the numerator is too low is greater than the underestimate when the numerator is, to the same extent, too high. A similar bias may be seen in comparing the mean of the reciprocals of a set of numbers, which is not the same as the reciprocal of their mean.

TABLE 5.1. - HYPOTHETICAL EXAMPLE OF ESTIMATED TOTAL INSTANTANEOUS MORTALITY COEFFICIENTS FOR DIFFERENT PAIRS OF YEAR AND AGE GROUPS

Age
Year	4/5	5/6	6/7	7/8	8/9	9+/10+	Mean
1950/51	0.75	0.80	0.65	0.70	0.55	0.75	0.70
1951/52	0.70	0.80	0.75	0.60	0.65	0.55	0.68
1952/53	0.65	0.60	0.85	0.70	0.85	0.70	0.72
1953/54	0.70	0.75	0.65	0.90	0.50	0.50	0.67
Mean	0.70	0.74	0.72	0.72	0.64	0.62	0.69

If there are no appreciable systematic differences between years or ages, then the entries in Table 5.1 can be considered as separate estimates of the same true mortality, and the combined estimate with least variance will be given by taking the weighted mean, with weights inversely proportional to the variances of the individual estimates. These variances will generally be unknown and though obviously the variance for the very old and scarce age groups will be high, the present evidence (on limited data for plaice, herring and haddock) suggests that the variance is about constant for all the well-represented age groups (from four to eleven years for the North sea plaice). Thus, if the data for the older groups are combined (e.g. the last column of Table 5.1), all the variances will be nearly equal, and the appropriate overall estimate will be the simple mean of all the individual estimates. The mean estimate for the mortality between any one pair of age groups in all years may be determined in the same way, i.e. taking the simple mean of the estimates of Z for each pair of years, or taking the weighted mean, giving greater weight to those years for which the variance of Z is likely to be small (e.g. years in which more samples were taken). Another common method for estimating the mortality between successive years is to take the decrease in the total numbers of all fully exploited year-classes, the ratio of four years and older to five years and older. This is effectively weighting the estimates in Table 5.1 by the numbers of fish at each age.

The effect of using poor figures for effort and catch per unit effort, such that the latter does not bear a constant ratio to the true abundance, may be deduced from equation (5.1). Suppose the relation between catch per unit effort and abundance in the two years is given, with the obvious notation, by

Then

and

,

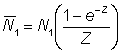
then

................. (5.3)

Thus the estimated mortalities will include an extra term, equal to the logarithm of the change in q (the "availability" or "catchability coefficient"). A change in q will show, in an array such as Table 5.1, as a year-to-year change in mortality unconnected with changes in fishing or other causes of mortality.

Changes in availability are particularly likely to cause serious errors when there is any trend in the values of q. This may be unlikely when using annual figures, but it is often tempting to use the decline in catch per unit effort (either of a single age group or of the stock as a whole) during part of the year to provide an estimate of mortality. Nearly all fisheries show more or less pronounced seasonal changes in catch per unit effort, but while in some fisheries the fluctuations in catches per unit effort may follow precisely the fluctuations in abundance - the increases being due to recruitment and growth, and the decreases due solely to mortality - very often the major causes of the fluctuations are "availability" changes, with the fish being particularly vulnerable to capture at certain seasons, e.g. when concentrated for spawning (similar fluctuations over a 24-hour cycle may often be observed, when there is no question that the decrease during, say, daylight, is due to the stock abundance really being lower).

As a general rule, therefore, short-term changes in catch per unit effort should not be used in estimating mortalities. Normally mortalities will be estimated from mean annual values, or from periods twelve months apart. Special techniques may be necessary to estimate indices of abundance and hence mortalities, for highly seasonal fisheries where the precise timing of the peak of the season may be different in different years.

Data on the abundance of successive year-classes at any one time can be used in a similar way as data on the abundance of the same year-classes in successive years. Taking t_c as the age at which fish enter the fishery, at which time the numbers of two successive year-classes are N₀, 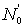, then their abundances at the time when they are aged t+ 1 and t will be

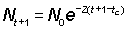
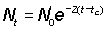
.............. (5.4)

Catch per unit effort, or even percentage age data, can be used as indices of abundance directly in the equation, for the coefficient q, related to availability, in the top and bottom of the ratio, relates to the same period, and therefore cancels out, namely

........... (5.5)

Equations (5.3) and (5.5) are very similar, both containing a term additional to the true mortality. Ratios of catch per unit effort of the same year-class, i.e. equation (5.3), are best to use when year-class strength is variable, or when "availability" changes are small and effort is readily measured. Equation (5.5) is better when measurement of effort is difficult, or year-class variation is small, and also when data are available only for isolated years. A disadvantage of equation (5.5) is that it does not measure the contemporary situation - the additional mortality undergone by the older of two adjacent age groups is that which it underwent during its first year in the fishery and later mortalities have been applied to both year-classes equally. Thus if fish recruit to the fishery at three years old, the ratio of six- to seven-year-old fish in the 1965 catches provides a measure of the mortality in 1961 (the year before the fish, now six years old, recruited to the fishery).

Estimates of total mortality over a period can also be obtained by semigraphical methods. If Z (the total mortality) is constant we can give the numbers of a year-class at any time, t, after it is subject to the full mortality, as

N_t =N₀e^-Zt

where

N₀ = numbers at time t = 0

therefore log_eN_t = log_e N₀ - Zt.

If now log_eN_t is plotted against t, it will give a straight line of slope - Z. This plot can be made either for the catch per unit of a single year-class at different times, in which case deviations (other than sampling variance) will be caused by changes in "availability," or for numbers of different year-classes at the same time - the well-known "catch-curve." (A detailed analysis of such curves, including the interpretation of nonlinear catch-curves is given by Ricker, 1958.) Deviations will be caused in the catch-curve by inequalities in original year-class strength, but the value of the "availability" in the year of sampling will alter only the position of the curve, and not its slope, or the scatter of the points. This graphical method can also (and probably most easily) be used by plotting N_t against t on semilogarithmic paper. This should give a straight line, from which the mortality is best estimated as follows. Note where the line has some convenient value (say 100), find the value on the line exactly one year later (equals say 65). Then the survival through one year 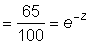from which Z can be at once looked up in suitable tables (see Figure 5.1).

FIGURE 5.1. - Estimation of Z. Plot of logarithm of abundance of a year-class against age.

All the above methods, when applied to age composition of catches, are subject to bias when the different ages are not equally vulnerable to the gear used. If the vulnerabilities of successive ages, t, t + 1 are _tq, _t+1q, the catches, or catches per unit effort, are given by

In practice this type of bias may be difficult to detect, unless there are abrupt changes with age, when the apparent mortality will also change with age, though the possibility of its existence should never be neglected. Because such differential vulnerability by age implies that fishing mortality is different for each age, the forms of the age distribution of the stock and of the age distribution of the catch may both be quite different from the simple constant mortality form (see Jones, 1964). Sometimes, e.g. when decreased vulnerability is balanced by decreased mortality, the age distribution of the catches may be very similar to that obtained with constant mortality.

Total mortality can also be estimated from the "virtual population." The virtual population of a certain year-class on say its eighth birthday is the total number of fish of that year-class caught after their eighth birthday.

The mortality during the ninth year of life is then obtained as the logarithm of the ratio of the virtual populations at nine and at eight years old. The method is probably most useful for long-lived fish, of which fishing takes a major share, but for which " availability" varies and is difficult to measure (see Fry, 1957; Bishop, 1959; Garrod, 1967).

When the age composition is not known, estimates, and sometimes quite good estimates, of total mortality can be obtained from length data. The relative number of fish in two different size groups will depend on (a) the time taken to grow from one size to the other, (b) the mortality rate during that period, and (c) the time taken to grow through each size group, i.e. the proportion each size group is of a complete year-class. These factors have one particularly simple case if there is a range of sizes over which the growth is linear, or approximately so. Then if the numbers in each size group are plotted on a logarithmic scale, as for a catch-curve, the plot should yield a straight line, whose slope is proportional to the total mortality coefficient. Further, if the growth rate is known, the absolute value of the mortality can be determined. The curve of growth in weight is generally more closely linear over a wider range of important sizes than is growth in length. Therefore a plot of numbers in each weight group against weight may provide a fair estimate of mortality. Mathematically if the growth curve over some range of age can be represented by the straight line l_t = a + bt and the slope of the plot of numbers against length is Z', i.e. 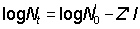, then Z = bZ', or if the growth in weight is linear so that W_t = C + gt, 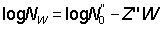, then Z = gZ".

The total mortality can also be measured in a steady state by the average age or length in the exploited population, which will, if the fishery is not selective, be the same as that in the catch. If the ages and lengths of entry into the exploited phase, and the averages in the catch, are t_c, l_c, 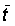, 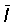respectively, the following expressions for Z can be readily derived (cf Beverton and Holt, 1956).

where K, L_¥ are the constants in the von Bertalanffy equation.

5.3 Fishing mortality

There are numerous methods for estimating the fishing mortality, none of which is entirely satisfactory, and in practice it is advisable to obtain estimates from as many independent methods as possible. The main methods are outlined here, dealing first with the more direct ones.

(a) Direct census. If the total stock is known, the fishing mortality can be calculated at once from the catch. The total numbers may be obtained by a complete or sample count, e.g. salmon passing upstream, or whales seen in a known area; usually, the only practicable method for marine fishes is the census of adult females by counting (by a suitable plankton survey) the number of eggs produced. The total numbers are then estimated from the proportion of adult females in the catch and their fecundity. An advantage is that it is rather easier than with most other methods to attach statistical confidence limits to the estimates, though these may be wide (see English, 1964).

(b) Swept area. From the definition of the instantaneous mortality coefficient it follows that the fishing mortality generated by a single operation, which may be considered as taking an infinitesimally small part of the whole stock, is equal to the fraction of the population which is caught. If the stock is evenly distributed and the gear effectively catches all the fish within a certain area, a, and the total area inhabited by the stock = A, then the fishing mortality is equal to 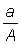. The mortality generated during a period of A. duration D f by the whole fishery, FD t, is then 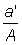, where a' is the sum of the areas covered by all the vessels of the whole fleet.
This method is, of course, confined to gear for which the area covered can be readily estimated, e.g. for a trawl haul the area covered would appear to be the distance between the doors times the distance covered. There are two important sources of error, acting in opposite directions. First, since some of the fish in the area covered by the gear may escape, the method may over-estimate the mortality. Secondly, as mentioned in the section on effort, the density of fish in the fished area will be greater than the average density, leading to an underestimate of the fishing mortality. The method therefore cannot give an exact estimate of fishing mortality, but may sometimes be useful to give an indication of its order of magnitude. In particular, it is often useful in showing whether fishing is likely to be having a significant effect. The method may also be used where the area covered by fish can be determined from an echo survey. (For uses of echo sounders, etc. in measuring abundance, and hence fishing mortality, see International Council for the Exploration of the Sea, 1964b, Symposium on the Measurement of the Abundance of Fish Stocks, especially papers by Cushing and Federov, and FAO, Report of ACMRR Working Party on Direct and Speedier Estimation of Fish Abundance, 1967.)

(c) Marking. Marking experiments are, of course, one of the best known methods of estimating the fishing mortality. If the population of marked fish is in all ways the same as the total stock, the fishing and total mortalities are readily determined from the number of marked fish returned. The methods are discussed in the next section.

(d) Changes in total mortality. If the amount of fishing changes, thus changing the fishing mortality, this will result in a change in total mortality. Thus if observed changes in the latter can be related quantitatively to known changes in fishing effort, the value of the fishing mortality coefficient at any given level of effort can be deduced. Mathematically, we have for a period of duration, T,

ZT = (F + M) T = qf + M,

where f is the total effort during the period.

If T = 1, then, using the data of effort during one year

Z = F + M = qf + M .................. (5.6)

or, in terms of the fishing intensity ;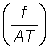

.

If a series of pairs of values of f (fishing effort) and Z (total mortality coefficient) are known, equation (5.6) gives a linear relation between them. For two such pairs this gives two simultaneous equations which can be solved for q and M. For more than two pairs the plot of Z against f should yield a straight line, slope q, and intercept on the y-axis M (see Figure 5.2).

FIGURE 5.2. - Estimation of F and M. Plot of mortality coefficient against fishing effort.

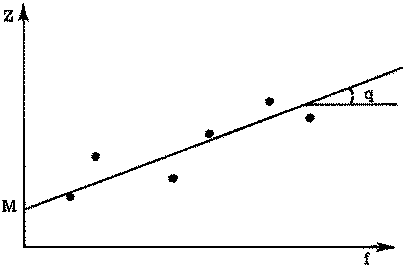
The solution for q and M can be obtained by the normal regression techniques of statistics, for equation (5.6) is in the standard form for a linear regression

y = a + bx

where y = total mortality coefficient: x = fishing effort.

Denoting by 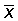, 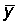the mean values of x and y, the least squares estimates for M and q are

.................. (5.7)

..................... (5.8)

The correlation coefficient, the significance level of the regression, and the confidence limits of M and q can be calculated by the standard techniques.

If the mortalities used in the regression have been estimated from indices of abundance at known instants in time, i.e. from equation (5.1), the results of the regression can be used at once. Thus the mortality between 1 January 1961 and 1 January 1962 can be related to the total fishing effort in 1961. More usually the indices of abundance will be known only as averages over a period - e.g. as the mean catches per unit effort in 1961 and in 1962. As equation (5.2) shows, the mortality estimated from the ratio of the two indices of abundance (i.e. 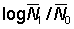) will be related to the mortalities (and hence fishing effort) in both periods. The mortality should therefore be related to the average of the total fishing effort in the two periods. Thus, for example, graphically we would plot the log ratio of the mean numbers in 1961 and 1962 against the average effort in 1961 and 1962. It can be shown that this method does in fact make the most efficient use of the data (Paloheimo, 1961).

This method, though most obviously used for effort varying with time, i.e. from year to year, can also be applied when the fishing effort varies with age, supposing that a selective gear is in commercial use, but that true density indices of each age group can be obtained, e.g. by special research vessel sampling.

When the estimates of mortality between successive pairs of years are highly variable, because of sampling errors or other causes (e.g. changes in "availability") rather than real changes in mortality, an average mortality coefficient over several years can be obtained by following the fortunes of a single year-class throughout its life in the exploited phase. If its density index is plotted on a logarithmic scale this should yield a straight line, slope equal to the mortality coefficient, and this last can be plotted against the average fishing effort in the corresponding period. Though this method gains in reducing the scatter, this is at the cost of losing information on the effect of year-to-year changes, though not of long-term trends, in fishing effort. Also, since the data for adjacent year classes come to a large extent from the same years, the points are not fully independent, and the exact tests of significance and confidence levels, outlined above, also cannot be applied.

5.4 Natural mortality and growth characters

An idea of the value of the natural mortality rate can be gauged from the growth pattern of the species concerned. A fish which approaches its ultimate length quickly - i.e. has a high value of K - is likely to have a high natural mortality, whereas a fish that grows slowly (a low K) is likely also to have a low M. The relation between M and K appears to differ from one group of fish to another - thus for clupeoids M is generally between one and two times K; for gadiforms M is generally between two and three times K. Like the swept area method for estimating fishing mortality this is not a precise method, but is often most useful in the early stages of studying a fishery in judging whether natural (or fishing) mortality is likely to be the dominant element in the total mortality (Beverton and Holt, 1959).

5.5 Special graphical methods

Graphical methods can also be used to estimate mortality rates from some other special situations where algebraic methods would be difficult. Regier (1962) has described some such situations where census data giving the stock in absolute numbers at different times are available and also statistics of total catch, either during a very short season (perhaps only a few days, so that fishing mortality is extremely high) or during a season covering only a part of the period between the two census dates.

FIGURE 5.3. - Graphical method for determining mortality rate.

Consider first a very short fishing season, during which 300 fish are caught in, say, two days. At some time, t₀, before the season say 1 000 fish were present, and at some time t₂ after the season say 300 fish were present. Using semilogarithmic paper, as in Figure 5.3, the population sizes at the two census dates are given by points A₀, A₂. If the mortality rates had been constant the population at the time of the fishing season, t₁, would have been A₁. In fact the real changes in the population would be described by the line A₀BCA₂ in the figure where BC represents the catch of 300 fish, and the two parallel lines A₀B, CA₂ the decrease due to natural mortality. As the two triangles A₀BA₁, A₁CA₂ are similar the ratio of the lengths BA₁: A₁C (as measured by a ruler on the semilogarithmic paper) will be equal to the ratio of the periods (t₂ - t₀): (t₂ - t₁). As a first approximation take some position of C and the position B at a value of C + 300. Measure with a ruler the distance BC (this distance will vary with the value of C). Then the distance CA₁ as measured with a ruler should be equal to 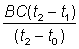. If it is greater than this the first approximation of C is too low; the correct value of C can thus quickly be found. The natural mortality is then readily determined from the line A₀B (or CA₂) - as usual this is most easily done by noting where it crosses the population size of 1, or 100 etc., and reading off the population size exactly one year later.

More generally, fishing takes place over a period - the open season - during which the fishing mortality can be taken as constant. Censuses are made at two times outside the fishing season, so that the time between the two censuses includes two periods before and after the open season during which only natural mortality is occurring. As a first approximation the known catch, C, may be assumed all to be taken at the midpoint of the season and, using the method described above, a first estimate of natural mortality, M₁, can be obtained. Using this value of M the deaths outside the fishing season can be calculated and hence, by subtraction, the total deaths N during the season, and the total mortality ZT (where T = duration of season) estimated.

Then F may be estimated from

Using this value in the equation 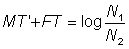, where N₁, N₂ are the numbers at the time of the two censuses, and T' the time between censuses, a second estimate, M₂, of natural mortality can be obtained. A third estimate of M is 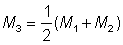, which can be used in the same way to obtain improved estimates of F and M. (The procedure of obtaining a succession of better and better estimates is called iteration.)

5.6 Exercises

1. (a) A certain mortality causes 25 percent of the population to die each year; what percentage of the initial population is left after 2 years; 6 months; 3 years? What is the corresponding instantaneous mortality coefficient? (N.B. find the coefficient first.) Repeat for mortalities causing 10 percent, 90 percent, 50 percent mortalities per year.

(b) Two causes of mortality, acting independently, cause mortality coefficients of 0.2 and 0.3; what is the resulting total mortality coefficient? Repeat with coefficients of 0.7 and 0.1; 1.0 and 0.3.

(c) Two causes of mortality act independently on a population; alone they would cause respectively 20 percent and 30 percent of the population to die within a year. Do 50 percent of the population die within a year? If not, what is the percentage that does die (N.B. convert to instantaneous mortality coefficients)? Repeat for pairs of mortalities causing 70 percent and 30 percent; 80 percent and 70 percent mortalities.

(d) Plot y against x, on semilogarithmic paper, and log₁₀y and log_ey against x on ordinary graph paper, for each set of y.

x ...................	0	1	2	3	4	5	6
y1 ...................	0.79	0.63	0.50	0.40	0.32	0.25	0.20
y2 ...................	0.71	0.35	0.18	0.089	0.045	0.022	0.011

Do the points satisfy the equation

log y = ax + b

or

y = c e^dx?

If so, what are the values of a, b, c, d? (Estimate these from the slope and intercept of the straight-line relation). If the y's are indices of the density of a certain year-class of fish at yearly intervals, do the data fit constant mortality coefficients? If so, what are the fractions surviving each year, and what are the instantaneous mortality coefficients?

2. A research vessel in five one-hour trawl hauls caught the following numbers of fish at each age

I, 30; II, 450; III, 120; IV, 70; V, 25; VI +, 15

A year later, the catches in twelve one-hour hauls were

I, 60; II, 960; III, 480; IV, 120; V, 72; VI +, 42

Assuming for the purposes of this example that, with the mesh size in use, the catch per hour gives a valid index of density for two-year-old fish and older, estimate the total mortality coefficient during the year. If the only data available were those for the first of these years, give an estimate of the average total mortality during the previous few years. (In practice 5 or 12 hauls would generally be quite insufficient to provide a valid index of density.)

3. A group of fish is subject in two successive years to total mortality coefficients of 0.85 and 0.80. If the number present at the beginning of the first year is 1 000 what is the average number present in each of the two years? What is the total mortality coefficient estimated from these two averages?

4. A plankton survey showed that 2 × 10¹¹ eggs were laid during a spawning season. Fecundity studies showed that the average mature female laid 10⁵ eggs. Market studies showed that 3 000 000 fish were landed during the year following, and of these 40 percent were adult females (i.e. had spawned at least once). What percentage of the spawning females was caught during the year? If the total mortality coefficient was 1.2, what were the fishing and natural mortality coefficients?

5. The average number of plaice landed at Lowestoft per 100 hours' fishing in two periods were

Age	2	3	4	5	6	7	8	9	10
Years
1929-38	125	1 355	2 352	1 761	786	339	159	70	28
1950-58	98	959	1 919	1 670	951	548	316	180	105

Calculate the average total mortality coefficients in the two periods. If the average total fishing efforts on plaice in the North sea during the two periods were, in terms of millions of hours fishing by United Kingdom steam trawlers, 5.0 in 1929-38, and 3.1 in 1950-58, estimate from equation (5.6) the natural mortality, and the fishing mortality in the two periods.

6. The table below gives the age composition of cod in terms of numbers landed per 100 ton-hours fishing by United Kingdom trawlers, in the Barents sea, and the total fishing effort in each year.

Age	3	4	5	6	7	8	9	10	11	12+	Effort
Year
1932	0.02	0.38	1.49	3.16	3.81	2.16	1.55	2.20	1.48	2.80	174
1933	-	0.37	2.12	3.37	4.62	3.37	1.60	1.37	0.64	2.18	184
1934	-	0.43	3.18	6.18	5.47	8.69	2.59	0.75	0.56	1.98	164
1935	0.03	0.56	7.85	13.97	10.04	5.82	2.82	0.88	0.29	1.22	184
1936	0.1	0.58	1.75	8.61	7.96	9.28	3.50	0.93	0.19	1.13	252
1937	0.02	1.89	2.91	7.66	17.05	8.65	2.53	0.38	0.26	0.33	321
1938	0.4	1.87	7.09	5.73	9.46	9.54	4.30	1.24	0.48	0.13	253
1946	7.3	11.52	11.39	18.91	13.77	11.77	14.29	17.65	5.26	3.13	66
1947	2.1	18.25	28.42	28.42	22.57	5.99	7.10	10.45	11.01	4.74	103
1948	0.9	1.89	15.59	33.19	26.77	11.31	7.17	2.01	3.65	2.76	156
1949	4.4	12.92	18.38	34.83	20.08	9.08	6.58	2.94	1.59	2.50	171
1950	-	0.49	1.15	5.49	12.89	9.32	4.28	1.76	1.01	0.37	248
1951	0.04	1.41	8.61	13.58	11.52	6.62	4.11	1.76	1.96	0.02	313
1952	0.15	4.65	11.84	16.26	13.14	4.09	2.11	1.52	0.91	0.93	412
1953	0.02	2.02	13.77	16.41	7.20	5.49	1.62	0.70	0.15	0.25	396
1954	0.07	5.33	19.03	21.76	20.25	3.99	1.62	0.48	0.67	0.13	425
1955	-	0.73	10.83	26.82	15.70	5.44	1.93	1.30	0.57	0.19	551
1956	0.02	0.50	6.29	22.76	13.51	5.59	1.20	0.61	0.24	0.08	630
1957	0.08	1.98	3.40	9.81	9.70	6.55	1.29	0.58	0.38	0.10	457
1958	0.6	4.4	9.98	8.31	5.81	5.53	1.56	0.91	0.42	0.20	414

Tabulate the total mortality coefficient for each pair of years for each age. Examine the data and decide from what age the fish are fully represented in the catches. What evidence is there that the age at full recruitment is different in the pre- and post-war periods? Hence obtain a single estimate for the mortality between successive pairs of years (take the ratio 7 and older; 8 and older). By relating this mortality graphically and by calculation of the regression line to the average fishing effort in the two years, estimate the natural mortality and the fishing mortality in 1958. What are the confidence limits to the natural mortality? (Note that the estimate of M obtained will, as usual, include any net migration from the fished area.)